Deviation From Ideal Gas Behavior

 

Table of Content

Causes of Deviation from Ideal Behaviour Van der Waals’ Equation of State for a Real Gas Volume Correction Pressure Correction Different forms of Van der Waal’s equation At Low Pressures At Fairly High Pressures At Very Low Pressures Hydrogen and Helium Some Other Important Definitions Relative Humidity (RH)  Boyle’s Temperature (Tb) Critical Temperature (Tc) Critical Pressure (Pc) Critical Volume Molar Heat Capacity of Ideal Gases Related Resources

The deviation from ideal behaviour is expressed by introducing a factor Z known as compressibility factor in the ideal gas equation. Z may be expressed as Z = PV / nRT   

In case of ideal gas, PV = nRT ∴ Z = 1 In case of real gas, PV ≠ nRt ∴ Z ≠ 1

Thus in case of real gases Z can be < 1 or > 1  

• When Z < 1, it is a negative deviation. It shows that the gas is more compressible than expected from ideal behaviour.  

• When Z > 1, it is a positive deviation. It shows that the gas is less compressible than expected from ideal behaviour. 

Causes of Deviation from Ideal Behaviour

The causes of deviations from ideal behaviour may be due to the following two assumptions of kinetic theory of gases. 

• The volume occupied by gas molecules is negligibly small as compared to the volume occupied by the gas.

• The forces of attraction between gas molecules are negligible.  

The first assumption is valid only at low pressures and high temperature, when the volume occupied by the gas molecules is negligible as compared to the total volume of the gas. But at low temperature or at high pressure, the molecules being in compressible the volumes of molecules are no more negligible as compared to the total volume of the gas.  

The second assumption is not valid when the pressure is high and temperature is low. But at high pressure or low temperature when the total volume of gas is small, the forces of attraction become appreciable and cannot be ignored.  

Example 1:

Question  1 mole of SO2 occupies a volume of 350 ml at 300K and 50 atm pressure. Calculate the compressibility factor of the gas.   Solution:  P = 50 atm       V = 350 ml = 0.350 litre   n = 1 mole    T = 300L  Z = PV / nRT     ∴ Z = 50 × 0.350 / 1 × 0.082 × 300 = 0.711     Thus SO2 is more compressible than expected from ideal behaviour.

Van der Waals’ Equation of State for a Real Gas

This equation can be derived by considering a real gas and 'converting ' it to an ideal gas.

Volume Correction

Hence, Ideal volume

 V_i = V – nb  …..........(i)

n = Number of moles of real gas

V = Volume of the gas  

b = A constant whose value depends upon the nature of the gas  

The Van der Waals constant b (the excluded volume) is actually 4 times the volume of a single molecule.

b = 4 × volume of a single molecule  = 4 ×  6.023 × 10²³ ×(4/3)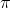r³, where r is the radius of a molecule.

Pressure Correction

Let us assume that the real gas exerts a pressure P. The molecules that exert the force on the container will get attracted by molecules of the immediate layer which are assumed not to be exerting pressure.

It can be seen that the pressure the real gas exerts would be less than the pressure an ideal gas would have exerted. The real gas experiences attractions by its molecules in the reverse direction. Therefore if a real gas exerts a pressure P, then an ideal gas would exert a pressure equal to P + p (p is the pressure lost by the gas molecules due to attractions).

This small pressure p would be directly proportional to the extent of attraction between the molecules which are hitting the container wall and the molecules which are attracting these.

Therefore p ∝ n/v (concentration of molecules which are hitting the container's wall)

P ∝ n/v (concentration of molecules which are attracting these molecules)   p ∝ n²/v²

P = an²/v² where a is the constant of proportionality which depends on the nature of gas. 

A higher value of 'a' reflects the increased attraction between gas molecules.

Hence ideal pressure  

P_i = (P + an² / V²)  …...........(ii)

Here,

n = Number of moles of real gas  

V = Volume of the gas  

a = A constant whose value depends upon the nature of the gas  

Substituting the values of ideal volume and ideal pressure in ideal gas equation i.e. pV=nRT, the modified equation is obtained as  

The constants a & b

Vander Waals constant for attraction (a) and volume (b) are characteristic for a given gas. Some salient feature of a & b are: For a given gas Vander Waal’s constant of attraction ‘a’ is always greater than Vander Waals constant of volume (b). The gas having higher value of ‘a’ can be liquefied easily and therefore H2 & He are not liquefied easily. The units of a = litre2 atm mole–2 & that of b = litre mole –1 The numerical values of a & b are in the order of 10–1 to 10–2 & 10–2 to 10–4 respectively.  Higher is the value of ‘a’ for a given gas, easier is the liquefaction.

Different forms of Van der Waal’s equation

• At Low Pressures

‘V’ is large and ‘b’ is negligible in comparison with V.

The Vander Waals equation reduces to:             

(P + a / V²) V = RT;         

PV + a/ V = RT            

PV = RT – a/V  or PV < RT            

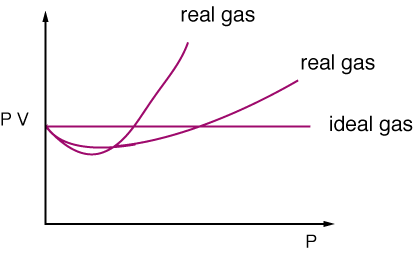
This accounts for the dip in PV vs P isotherm at low pressures.

• At Fairly High Pressures

a/V²  may be neglected in comparison with P.

The Vander Waals equation becomes            

P (V – b) = RT

PV – Pb = RT            

PV = RT + Pb or PV > RT            

This accounts for the rising parts of the PV vs P isotherm at high pressures.

• At Very Low Pressures

V becomes so large that both b and a/V² become negligible and the Vander Waals equation reduces to PV = RT. This shows why gases approach ideal behaviour at very low pressures.
 

• Hydrogen and Helium

These are two lightest gases known. Their molecules have very small masses. The attractive forces between such molecules will be extensively small.  So a/V² is negligible even at ordinary temperatures. 

Thus PV > RT. Thus Vander Waals equation explains quantitatively the observed behaviour of real gases and so is an improvement over the ideal gas equation.  Vander Waals equation accounts for the behaviour of real gases. 

At low pressures, the gas equation can be written as,   

(P + a/v²_m) (V_m)  = RT

or

Z = V_m / RT = 1 – a/V_mRT  

Where Z is known as compressibility factor. Its value at low pressure is less than 1 and it decreases with increase of P. For a given value of V_m, Z has more value at higher temperature.  

At high pressures, the gas equation can be written as  

P (V_m – b) = RT  

Z = PV_m / RT = 1 + Pb / RT  

Here, the compressibility factor increases with increase of pressure at constant temperature and it decreases with increase of temperature at constant pressure. For the gases H₂ and He, the above behaviour is observed even at low pressures, since for these gases, the value of ‘a’ is extremely small.  

Example 2:

Question  One litre of a gas at 300 atm and 473 K is compressed to a pressure of 600 atm and 273 K. The compressibility factors found are 1.072 & 1.375 respectively at initial and final states. Calculate the final volume.   Solution:  P1V1 = Z1nRT1 and P2V2 = Z2nRT2   P2V2 / T2 × T1 / P1V1 = Z2 / Z1    or V2 =  Z2 / Z1 × T1 / T1 × P1V1 / P2  = 1.375 / 1.072 × 273 / 473  × 300 × 1 / 600 = 370.1 ml

Some Other Important Definitions
 

• ​Relative Humidity (RH)  

At a given temperature it is given by equation  

RH = (partial pressure of water in air) / (vapour pressure of water )
 

• Boyle’s Temperature (Tb)

Temperature at which real gas obeys the gas laws over a wide range of pressure is called Boyle’s Temperature. Gases which are easily liquefied have a high Boyle’s temperature [T_b(O₂)] = 46 K] whereas the gases which are difficult to liquefy have a low Boyle’s temperature [T_b(He) = 26K]. 

Boyle’s temperature(T_b)  = a / Rb = 1/2 T₁  

where T_i is called Inversion Temperature and a, b are called van der Waals constant.  

• Critical Temperature (T_c)

It (T_c) is the maximum temperature at which a gas can be liquefied i.e. the temperature above which a gas can’t exist as liquid.  

T = 8a / 27Rb

• Critical Pressure (P_c)

It is the minimum pressure required to cause liquefaction at T_c  

P_c = a/27b²  

• Critical Volume

It is the volume occupied by one mol of a gas at T_c and P_c  

V_c = 3b  

Molar Heat Capacity of Ideal Gases

Specific heat c, of a substance is defined as the amount of heat required to raise the temperature of is defined as the amount of heat required to raise the temperature of 1 g of substance through 1⁰C, the unit of specific heat is calorie g^-1 K^-1. (1 cal is defined as the amount of heat required to raise the temperature of 1 g of water through 1⁰C)  

Molar heat capacity C, is defined as the amount of heat required to raise the temperature of 1 mole of a gas through 1⁰C. Thus,  

Molar heat capacity = Sp. Heat  molecular wt. Of the gas  

For gases there are two values of molar heats, i.e., molar heat at constant pressure and molar heat at constant molar heat at constant volume respectively denoted by C_p and C_v. C_p is greater than C_v and C_p-R = 2 cal mol^-1 K^-1 

From the ratio of C_p and C_v, we get the idea of atomicity of gas.  

For monatomic gas C_p = 5 cal and C_v =3 cal  

∴ (γ = 5/3 = 1.67    (γ is poisson's ratio = C_p / C_v)    

For diatomic gas C_p = 7 cal and C_v = 5 cal

 γ = 7/5 = 1.40

For polyatomic gas C_p = 8 cal and C_v= cal  

γ   = 8/6 = 1.33

also C_p = C_pm,  

where, C_p and C_v are specific heat and m, is molecular weight.   

Example 3:

Question  Calculate Vander Waals constants for ethylene   TC = 282.8 k; PC = 50 atm   Solution:   b = 1/8 RTc / Pc = 1/8 × 0.082 × 282.8 / 50 = 0.057 litres/mole    a = 27 / 64 R2 × T2C / Pc = 27/64 × (0.082)2 × (282.8)2 × 1/50 = 4.47 lit2 atm mole–2

Question 1: When Z < 1 , it showns

a. a negative deviation

b. a positive deviation.

c. that the gas is showing ideal behaviour

d. that the gas is less compressible than expected from ideal behaviour

Question 2: A real gas behaves ideally at 

a. high temperature and low pressure

b. high temperature and high pressure

c. low temperature and high pressure

d. low temperature and low pressure

Question 3: Which of the following equations represents the correct form of Vandear waal’s equation at very low pressure? 

a. PV = RT – a/V

b. pV = nRT

c. P (V_m – b) = RT  

d. P (V – b) = RT  

Question 4: The maximum temperature at which a gas can be liquefied is called

a.boiling point.

b. melting point

c. Boyle’s temperature

d. critical temperature

Q.1	Q.2	Q.3	Q.4
a	a	b	d

Related Resources

• Click here to go through JEE Chemistry Syllabus

• Look here for Reference books for IIT JEE 

• You can also refe to the Ideal Gas Law & Dalton’s Law of Partial Pressure 

To read more, Buy study materials of States of Matter comprising study notes, revision notes, video lectures, previous year solved questions etc. Also browse for more study materials on Chemistry here.