Find the minimum value of given expressionWhat is the answer of given question
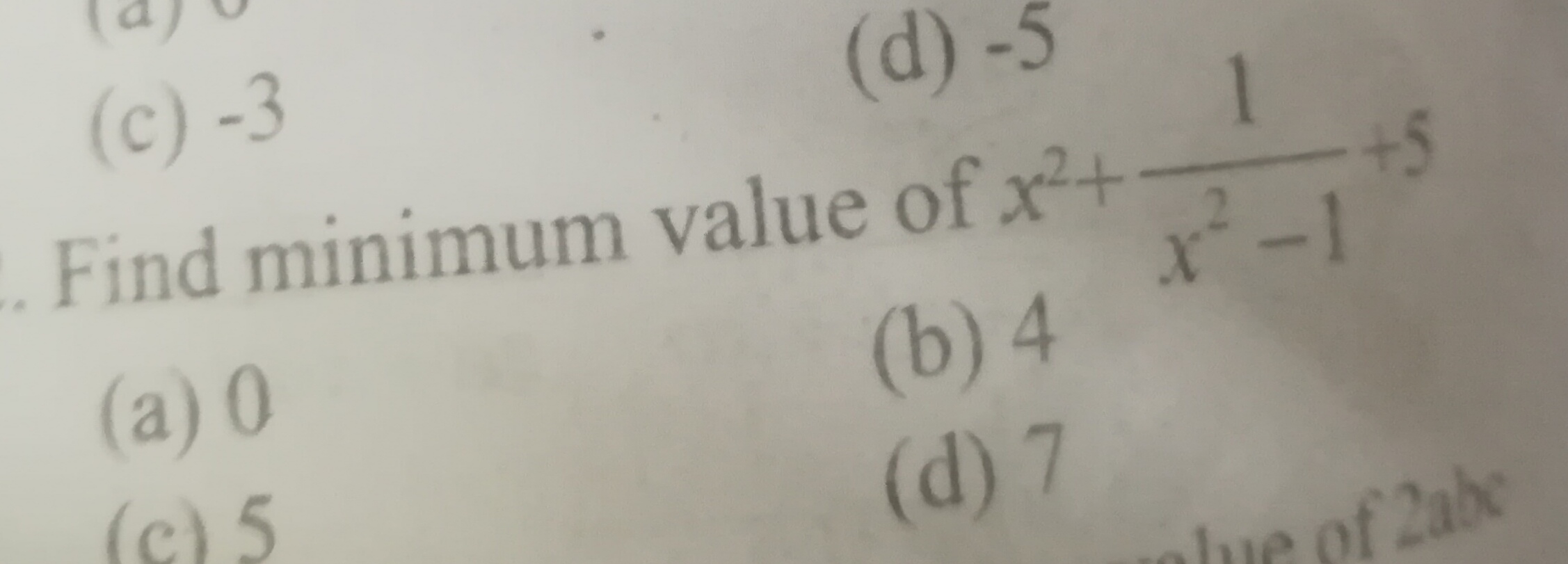
 10 grade maths> Find the minimum value of given expressio...
10 grade maths> Find the minimum value of given expressio...
 1 Answers
1 Answers
Last Activity: 6 Years ago

Prepraring for the competition made easy just by live online class.

Full Live Access

Study Material

Live Doubts Solving

Daily Class Assignments
Get your questions answered by the expert for free

Last Activity: 1 Year ago

Last Activity: 2 Years ago

Last Activity: 2 Years ago

Last Activity: 2 Years ago

Last Activity: 2 Years ago