how to solve inequalities which have more than one equal roots in numerator or denominator by plotting them on number line

eg: (-2x-10) (3-x)

------------------ < 0

(x^2+5)(x-2)^2

sakthi priya amirtharaj, 12 years ago

Grade:11

1 Answers

Aman Bansal

592 Points

12 years ago

Dear Sakthi,

We can use the number line to solve inequalities containing < , ≤ , > , and ≥ . To solve an inequality using the number line, change the inequality sign to an equal sign, and solve the equation. Then graph the point on the number line (graph it as an open circle if the original inequality was "<" or ">"). The number line should now be divided into 2 regions -- one to the left of the point and one to the right of the point

Next, pick a point in each region and "test" it -- see if it satisfies the inequality when plugged in for the variable. If it satisfies the inequality, draw a dark line from the point into that region, with an arrow at the end. This is the solution set to the equation: if one point in the region satisfies the inequality, the entire region will satisfy the inequality.

Example: -3(x - 2)≤12

Solve -3(x - 2) = 12 :

x - 2 = - 4
x = - 2

Graph x = - 2 , using a filled circle because the original inequality was ≤ :

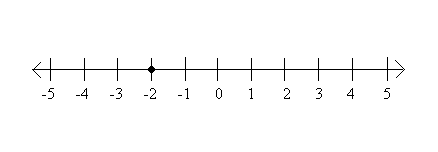
Graph of x = - 2

Plug values into the equation -3(x - 2)≤12 :

Pick a point on the left of -2 ( -3 , for example):
-3(- 3 - 2)≤12 ?
15≤12 ? No.
Pick a point on the right of -2 ( 0 , for example):
-3(0 - 2)≤12 ?
6≤12 ? Yes.

Draw a dark line from -2 extending to the right, with an arrow at the end:

Graph of -3(x - 2)≤12 , or of x≥ - 2

Thus, x≥ - 2

Best Of luck

Cracking IIT just got more exciting,It s not just all about getting assistance from IITians, alongside Target Achievement and Rewards play an important role. ASKIITIANS has it all for you, wherein you get assistance only from IITians for your preparation and win by answering queries in the discussion forums. Reward points 5 + 15 for all those who upload their pic and download the ASKIITIANS Toolbar, just a simple to download the toolbar….

So start the brain storming…. become a leader with Elite Expert League ASKIITIANS

Thanks

Aman Bansal

Askiitian Expert