is there anyone who can explain me the completing the square method in quadratic equation............

Dear Deepak,

First recall the algebraic identities

We shall use these identities to carry out the process called Completing the Square. For example, consider the quadratic function

What can be added to yield a perfect square? Using the previous identities, we see that if we put 2e=8, that is e=4, it is enough to add 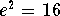to generate a perfect square. Indeed we have

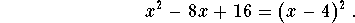
It is not hard to generalize this to any quadratic function of the form 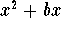. In this case, we have 2e=b which yields e=b/2. Hence

Example: Use Complete the Square Method to solve

Solution.First note that the previous ideas were developed for quadratic functions with no coefficient in front of . Therefore, let divide the equation by 2, to get

which equivalent to

In order to generate a perfect square we add 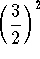to both sides of the equation

Easy algebraic calculations give

Taking the square-roots lead to

which give the solutions to the equation

We have developed a step-by-step procedure for solving a quadratic equation; or, in other words, an algorithm for solving a quadratic equation. This algorithm can be stated as a formula called Quadratic Formula

Please feel free to post as many doubts on our discussion forum as you can. If you find any question
Difficult to understand - post it here and we will get you the answer and detailed solution very quickly. We
are all IITians and here to help you in your IIT JEE preparation.


All the best Deepak !!!




Regards,

Askiitians Experts

Rohith Gandhi P