Please tell above answer quickly and as soon as possible.........................
Anvita Mahajan , 5 Years ago
Grade
Algebra> Please tell above answer quickly and as s...
1 AnswersSamyak Jain
Last Activity: 5 Years ago
|x| is always positive irrespective of sign of x.
|x + a| = x + a for x + a 
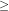
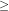
0 i.e. x –a & |x + a| = –(x + a) for x + a
Given : x, |x+1|, |x–1| are first three terms of an AP. 
2|x+1| = x + |x–1|.
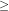
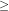
Case 1. Let x 1. Then x+1 > 0 & x – 1 0 i.e. |x+1| = x+1 & |x – 1| = x – 1
So, 2|x+1| = x + |x–1| 
2(x+1) = x + x – 1 2 = – 1, which is false.
Case 2. Let –1 
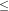
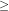
x 0 & x – 1
So, 2|x+1| = x + |x–1| 2(x+1) = x – (x – 1) 2x + 2 = x – x + 1 or 2x = – 1
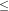
i.e. x = – 1/2. Clearly x = – 1/2 satisfies –1 x
|x+1| = x + 1 = – 1/2 + 1 = 1/2 and |x – 1| = – (x – 1) = – (–1/2 – 1) = 3/2
Sum of first n terms of an AP is (n/2)[2a + (n – 1)d].
= 180
Case 3. Let x – 3/2, 1/2 , 5/2 are the first 3 terms. Common diference d is 1/2 – (–3/2) = 2 and first term a is –3/2. sum of first 20 terms of the AP is (20/2)[2.(–3/2) + (20 – 1).2] = 10[ –3 + 38] = 10(35)
So, 2|x+1| = x + |x–1| – 2(x+1) = x – (x – 1) – 2x – 2 = x – x + 1 or – 2x = 3
i.e. x = – 3/2. Clearly x = – 3/2 satisfies x
|x+1| = – (x + 1) = – (–3/2 + 1) = 1/2 and |x – 1| = – (x – 1) = – (–3/2 – 1) = 5/2
= 350
Thus, the sum of first 20 terms of the AP is 180 or 350.
Provide a better Answer & Earn Cool Goodies
Enter text here...
LIVE ONLINE CLASSES
Prepraring for the competition made easy just by live online class.
Full Live Access
Study Material
Live Doubts Solving
Daily Class Assignments
Ask a Doubt
Get your questions answered by the expert for free
Enter text here...
Other Related Questions on Algebra
Can we divide two vectors? If this is possible then how?
Algebra
1 Answer Available
Last Activity: 10 Month ago(s)
How do you write “the product of 18 and q” as an algebraic expression?
Algebra
1 Answer Available
Last Activity: 10 Month ago(s)
दहावीच्या आय सी एस इ विद्यार्थ्यांनी पुढे काय करायला पाहिजे
Algebra
0 Answer Available
Last Activity: 1 Year ago(s)