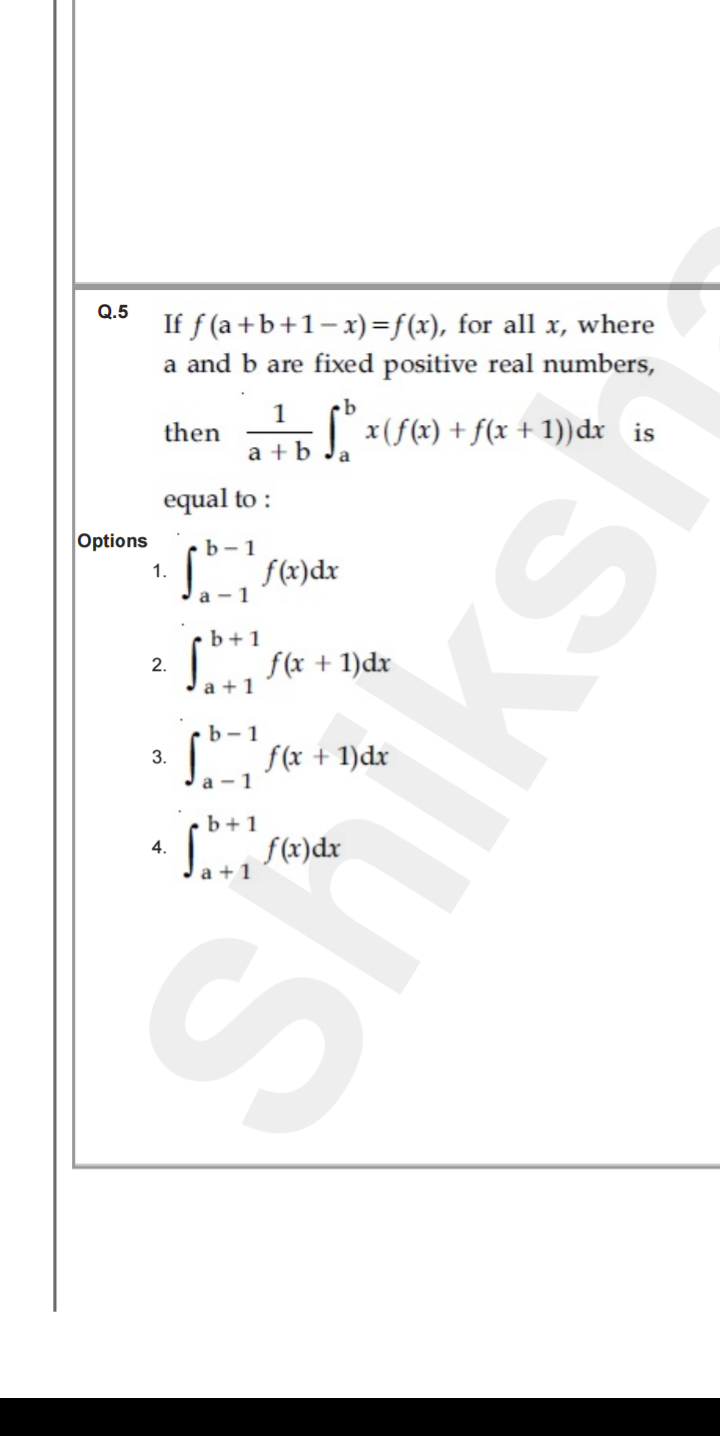
Kindly show me the solution for the given sum. It is taken from the January session of the JEE main examinations.
Get your questions answered by the expert for free

Last Activity: 2 Year ago(s)

Last Activity: 2 Year ago(s)

Last Activity: 2 Year ago(s)

Last Activity: 2 Year ago(s)

Last Activity: 2 Year ago(s)