Examples on Definite Integral - Study Material for IIT JEE

Calculus
OFFERED PRICE: Rs. 1,696
View Details

Objective Problems of Definite Problems



Objective Problems

Q1.   

(A)  0

(B) 100

(C)  200

(D) 400

Solution   




= –200 (cos x)^π₀= –200 (–1–1) = 400

 


Q2. ∫²_–2 min (x–[x], – x – [–x])dx,   where [.] is the greatest integer function =

(A)  0

(B) 1

(C) 2 

(D) None of these.

 

Solution:

Let I = ∫²_–2 min (x – [x], – x – x [–x])dx

= 2 ∫²_–2 min (x – [x], – x – [–x])dx (function is even)





= 1/4 + 1/4 + 1/4 + 1/4 = 1

Hence I = 1



 

Q3. (where [.] denotes greatest integer function) =

(A)  2-√2 

(B)  2+√2

(C)  2√2 

(D) √2

Solution: 



 

= (√2 – 1) + 2 (3/2 – √2) = √2 – 1 + 3 – 2 √2 = 2 + √2 – 2 √2 = 2 – √2

 

Q4. Q^p/2₀ sin 2 x in tan x dx = 

(A)  2 

(B) 0

(C) 1 

(D) None of these.

Solution:

I = ∫^π/2₀ sin 2x ln (tan x) dx      … (1)

I = ∫^π/2₀ sin 2 (π/2 – x) ln tan (π/2 – x)dx

I = ∫^π/2₀ sin 2x ln (cot x) dx     … (2)

Adding (1) and (2)

2I = ∫^π/2₀ sin 2x [ln (tan x) + ln (cot x)] dx = ∫^π/2₀ sin 2x ln 1 dx = 0.

  

Q5. O²_1/2 1/x sin e/e x – 1/x o/o dx =

(A)  0 

(B)  1

(C)  2 

(D)  √2

Solution: 

Let I = 

Put z = x - 1/x

Then I =  is odd function

  

Q6. The value of ∫^π/2₀ √sin x / √sin x + √cos x is

(A) p/2 

(B) p/3

(C) π/4  

(D) p

Solution:

Let I = ∫^π/2₀ √sin x / √sin x + √cos x dx 

Here the lower limit is zero hence , we can replace   x by (a – x) i.e. by p/2 –x



 

Q7.    =

(A) 3/p log 2 

(B) 2/p log 2

(C) 3/p log 3 

(D) 3/p

Solution: 



r/n = x, 1/n = dx

  

 

Q8. The area bounded by the curve y = x (3 – x)² , the x – axis and the ordinates of the maximum and minimum points of the curve is

(A) 2 sq. units 

(B) 6 sq. units

(C) 4 sq. units  

(D) 8 sq. units

Solution:

y = x (3 – x)²

After solving , we get x =1 and x = 3 are points of maximum and minimum respectively.

Now the shaded region is the required region



  



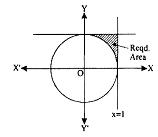
Q9. What is the area of a plane figure bounded by the points of the lines max (x, y) = 1 and x² + y² = 1 ?

(A) p/2 sq. units 

(B) p/3 sq. units

(C) p/4  sq. units 

(D) p sq. units

Solution:

By definition the lines max, (x, y) = 1 means.

x = 1 and y < 1 or y = 1 and x < 1

Required area                       





 

Q10. The area bounded by the curve y = (x –1) (x – 2) (x – 3) lying between the ordinates x = 0 and x = 3 is

(A) 7/4 sq. units 

(B) 4 sq. units

(C) 11/ 4 sq. units 



(D) 3 sq. units

 

 = 9 / 4 + 1/ 4 + 1/ 4 = 11/4  sq units.