Factors Affecting Rate of Reaction

Table of Content

Nature of the Reactants
Concentration of Reactants
Effect of Temperature
- Notable Points
Arrhenius Equation
Catalyst
General Characteristics of Catalyst
- Presence of a Positive Catalyst
- Presence of Negative Catalyst
Related Resources

Nature of the Reactants

(i) Physical State of Reactants

This has considerable effect over rate of reaction.
Decreasing rate of reaction: Gaseous state> Liquid state>Solid state
In a heterogeneous system collision is not so effective as in homogenous system. Thus, reactions in liquid phase or solution phase will be faster in comparison to heterogeneous conditions when same concentrations of the reactants are taken.

(ii) Physical Size of the Reactants

Among the solids, rate increases with decrease in particle size.
In powdered state rate of reaction is maximum because in powdered state, surface area is maximum.

(iii) Chemical Nature of Reactants

Consider the following two reactions:

2NO(g) + O₂(g) → 2NO₂(g) ...... (i)

CH₂(g) + 2O₂(g) → CO₂(g) + 2H₂O ..... (ii)

The first reaction is faster than the second because in the first reaction only N ≡ O bond is to be broken where as in the second reaction four (C-H) bonds are to be broken.
Similarly consider another example of two similar reactions:

2NO(g) + O₂(g) → 2NO₂(g) ...... (iii)

2CO(g) + 2O₂(g) → 2CO₂(g) ...... (iv)

NO bond is weaker than CO bond, hence broken easily. Thus reaction (iii) is faster than (iv).

Concentration of Reactants

Let us consider the reaction:

A + B → C + D; Rate = k[A][B]

Rate of the above reaction decreases with the passage of time because the concentration of reactants A and B will decrease as time pass on.
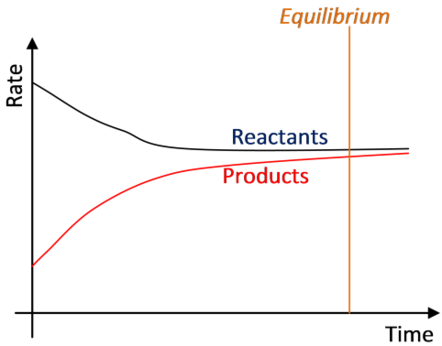
Let us consider the following reversible reaction: A + B ↔ C + D
Rate of forward reaction = k_f[A][B]
Rate of backward reaction = k_b[C][D]
Rate of forward reaction decreases and that of backward reaction increases with passage of time.
At equilibrium both the rates become equal.

Refer to the following video for factors affecting rate of reaction

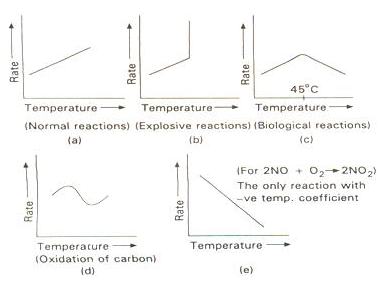
Effect of Temperature

The rate of reaction increases considerably with an increase in temperature.
The rates of many reactions are approximately doubled or tripled for every 10^oC rise in temperature.
The temperature coefficient of a chemical reaction is defined as the ratio of the specific reaction rates of a reaction at two temperature differing by 10^oC.
μ = Temperature coefficient= k_(r+10)/k_t
Let temperature coefficient of a reaction be ' μ ' when temperature is raised from T₁to T₂; then the ratio of rate constants or rate may be calculated as

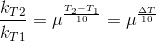
$\frac{k_T_2}{k_T_1}=\mu ^\frac{{T_2-T_1}}{10} =\mu ^{\frac{\Delta T}{10}}$

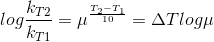
$log\frac{k_T_2}{k_T_1}=\mu ^\frac{{T_2-T_1}}{10} =\Delta T log\mu$

$\frac{k_T_2}{k_T_1}= antilog[\frac{\Delta T}{10 }] log\mu$

Its value lies generally between 2 and 3.

In order to explain the effect of temperature on the reaction rate. Arrhenius proposed a theory of reaction rate which states as follows :

A chemical reaction takes place by collision between the reactant molecules, and collision to be effective the colliding molecules must posses some certain minimum energy called threshold energy of the reaction.
Reactant molecules having energy equal or greater than the threshold are called active molecules and those having energy less than the threshold are called passive molecules.
At a given temperature there exists a dynamic equilibrium between active and passive molecules. The process of transformation from passive to active molecules being endothermic, increase of temperature increases the number of active molecules and hence the reaction.

Passive molecules $\rightleftharpoons$ Active molecules, DH = +ve

Concept of energy of activation (Ea)
The extra amount of energy which the reactant molecules (having energy less than the threshold) must acquire so that their mutual collision may lead to the breaking of bond(s) and hence the reaction, is known as energy of activation of the reaction. It is denoted by the symbol E_a. Thus,

E_a = Threshold energy – Actual average energy

E_a is expressed in kcals mole^–1 or kJ mole^–1.

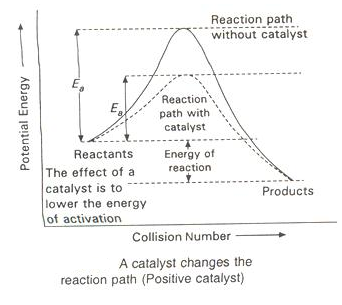
The essence of Arrhenius Theory of reaction rate is that there exists an energy barrier in the reaction path between reactant(s) and product(s) and for reaction to occur the reactant molecules must climb over the top of the barrier which they do by collision. The existence of energy barrier and concept of Ea can be understood from the following diagram.

? $\sum H_R$ = Summation of enthalpies of reactants

$\sum H_P$ ?= Summation of enthalpies of products

$\Delta$ H = Enthalpy change during the reaction

Ea₁ = Energy of activation of the forward reaction (FR)

Ea₂ = Energy of activation of the backward reaction (BR)

Notable Points

dH = Energy activation of FR – Energy of activation of BR
In the diagram mentioned above, FR is exothermic (dH is –ve) while BR is endothermic (dH is +ve). The minimum activation energy of FR i.e. any exothermic reaction will be zero while minimum activation energy for BR i.e. any endothermic reaction will be equal to DH.
Greater the height of energy barrier, greater will be the energy of activation and more slower will be the reaction at a given temperature.
Rate = Collision frequency $\times$ fraction of the total number of collision which is effective.
Rate = Collision frequency $\times$ fraction of the total number of collisions in which K.E. of the colliding molecules equals to Ea or exceeds over it.

Collision frequency is the number of collisions per unit volume per unit time. It is denoted by the symbol Z. Z is directly proportional to ÖT. By 10° rise in temperature. So, it is the fraction of the total number of effective, collisions that increases markedly by 10° rise in temperature resulting into marked increase in the reaction rate.

Arrhenius Equation

When the temperature is increased, heat energy is supplied which increases the kinetic energy of the reacting molecules.
This will increase the number of collisions and ultimately the rate of reaction will be enhanced. Arrhenius suggested an equation which describes k as a function of temperature, i.e.,
k = Ae^-E_a/RT
where k → rate constant

A → a constant (frequency factor)

E_a → energy of activation

At two temperature T₁ and T₂, taking log of Arrhenius equation, we get

log_e k₁ = log A - E_a/RT₁ log_e e .... (i)

and log_e k₂ = log A - E_a/RT₂ log_e e .... (ii)

subtracting Eq. (ii) from Eq. (i) and converting the log to the base 10, we get

log₁₀k₁/k₂ = -E_a/(2.303 R) [(T₂-T₁)/(T₁ T₂)]
or

$log\frac{k_2}{k_1} =\frac{E_a}{2.303R}[\frac{1}{T_1}-\frac{1}{T_2}]$

This equation can be used for calculation of energy of activation.

Logarithmic Arrhenius equation is:

log₁₀ k = log₁₀ A–E_a/(2.303 R) [1/T]
Y = C + M X
It is the equation of straight line with negative slope. On plotting log₁₀ k against [1/T] we get a straight line. The graph gives two kinetic parameters.
The slope gives activation energy and intercept gives frequency factor.
Reactions on the basis of influence of temperature are classified into five types.

Catalyst

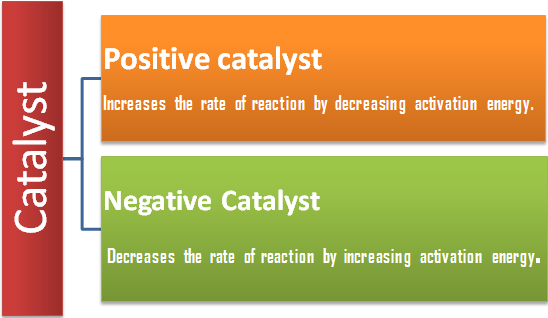
A catalyst is a substance, which increases the rate of a reaction without itself being consumed at the end of the reaction, and the phenomenon is called catalysis. There are some catalysts which decrease the rate of reaction and such catalysts are called negative catalyst. Obviously, the catalyst accelerating the rate will be positive catalyst. However, the term positive is seldom used and catalyst itself implies positive catalyst.

Catalyst are generally foreign substances but sometimes one of the product formed may act as a catalyst and such catalyst is called “auto catalyst” and the phenomenon is called auto catalysis.

General Characteristics of Catalyst

A catalyst does not initiate the reaction. It simply fastens it.
Only a small amount of catalyst can catalyse the reaction.
A catalyst does not alter the position of equilibrium i.e. magnitude of equilibrium constant and hence dG⁰. It simply lowers the time needed to attain equilibrium. This means if a reversible reaction in absence of catalyst completes to go to the extent of 75% till attainment of equilibrium, and this state of equilibrium is attained in 20 minutes then in presence of a catalyst also the reaction will go to 75% of completion before the attainment of equilibrium but the time needed for this will be less than 20 minutes.
A catalyst drives the reaction through a different route for which energy barrier is of shortest height and hence Ea is of lower magnitude. That is, the function of the catalyst is to lower down the activation.

Presence of a Positive Catalyst

The function of a positive is to lower down the activation energy.
The greater the decrease in the activation energy caused by the catalyst, higher will be the reaction rate.
In the presence of a catalyst, the reaction follows a path of lower activation energy. Under this condition, a large number of reacting molecules are able to cross over the energy barrier and thus the rate of reaction increases.
Activation energy is lowered in presence of a catalyst.

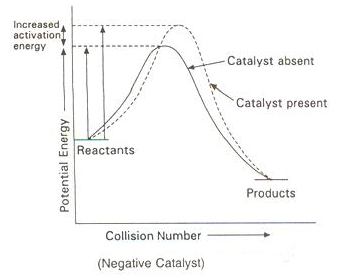
Presence of Negative Catalyst

A negative catalyst increases the activation energy of reaction by forming a new intermediate of high energy, i.e., by changing the reaction mechanism.
Due to increased activation energy, some active molecules become inactive, therefore, rate of reaction decreases.

Let 'p' denote presence of catalyst and 'a' denote absence of catalyst.

k_p = Ae^-E_p/RT .... (i)

k_a = Ae^-E_a/RT ... (ii)

Dividing (1) by (2), we get

k_p/k_a = e^{(E_a - E_p )/RT} = e^?E/RT

k_p/k_a = Antilog[?E/(2.303 RT)]

Solved Example

Example : The rate of a reaction triples when temperature changes from 20^oC to 50^oC. Calculate energy of activation for the reaction (R = 8.314 JK^-1 mol^-1).

Solution:

The Arrhenius equation is

log₁₀k₂/k₁ = E_a/(R×2.303) [(T₂-T₁)/(T₁ T₂ )]

Given: k²/k¹ = 3; R=8.314 JK^-1 mol–1; T1 = 20 + 273 = 293 K

and T₂ = 50 + 273 = 323 K

Subtracting the given values in the Arrhenius equation,

log₁₀ 3 = E_a/(8.314×2.303) [(323-293)/(323×293)]

E_a = (2.303 × 8.314×323 × 293×0.477)/30

= 28811.8 J mol^-1

= 28.8118 kJ mol^-1

Example :

In Arrhenius equation for a certain reaction, the value of A and E_a(activation energy) are 5×1013 sec^-1 and 98.6 kJ mol^-1 respectively. At what temperature, the reaction will have specific rate constant 1.1 × 10^-3 sec^-1 ?

Solution :

According to Arrhenius equation

k = Ae^-E_a/RT

or log_e k = log_e A - E_a/RT log_e e

or 2.303 log10 k = 2.303 log10 A - E_a/RT

or 2.303 log (1.1×10^-3)

= 2.303 log(4×10¹³)-(98.6×10³)/(8.314×T)

T = (98.6×10³)/(8.314×2.303×16.56) K

= 310.96 K

Example :

The rate constant is given by Arrhenius equation

k = Ae^-E_a/RT

Calculate the ratio of the catalyst and uncatalysed rate constants at 25^oC if the energy of activation of a catalysed reaction is 162 kJ and for the uncatalysed reaction the value is 350 kJ.

Solution :

Let k_ca and k_un be the rate constants for catalysed and uncatalysed reactions.

2.303 log₁₀ k_ca = 2.303 log₁₀ A - (162×10³)/RT .... (i)

and 2.303 log₁₀ k_un = 2.303 log₁₀ A - (350 × 10³)/RT .... (ii)

Subtracting Eq. (ii) from Eq. (i)

log₁₀k_ca/k_un = 10³/(2.303 RT) (350-162)

= (188× 10³)/(2.303×8..314×298) = 32.95

k_ca/k_un = 8.88 × 10³²

Question 1:

Which of the following factors does not affect rate of a reaction ?

a. Temperature

b. Catalyst

c. Concentration of reactants

d. Nature of Product

Question 2:

Among the solids, rate increases with decrease in particle size.

a. pressure

b. particle size

c. concentration

d. mass of particle

Question 3:

A negative catalyst increases

a. rate of reaction

b. activation energy

c. amount of product formed

d. rate of backward reaction

Question 4:

The slope of plot log₁₀ k vs [1/T] gives activation energy and intercept gives frequency factor

a.frequency factor

b. rate constant

c.rate f reaction

d.temperature coefficient

Q.1	Q.2	Q.3	Q.4
d	b	b	a

Related Resources

Click here for the detailed Syllabus of IIT JEE
Look here for Useful Books of Chemistry for IIT JEE
You can also refer to Introduction to chemical kinetics

To read more, Buy study materials of Chemical Kinetics comprising study notes, revision notes, video lectures, previous year solved questions etc. Also browse for more study materials on Chemistry here.