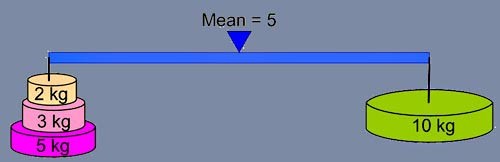
Relationship between A.M. and G.M.
Table of contents |
Arithmetic Mean
Arithmetic Mean is the average of two given numbers. If we have two numbers a and b, then we can include a number A in between these numbers so that the three numbers will form an arithmetic sequence like a, A, b.
Here the number A is the arithmetic mean of the numbers a and b.
According to the property of Arithmetic progression, we can say that-
A – a = b – A that is, the common difference ‘d’ of the given AP.
This is generally used to find the missing number of the sequence between the two given numbers.
The arithmetic mean serves as the balancing point of the two given numbers.
Example
What is the Arithmetic mean of 4 and 16 ?
Given
a = 4
b = 16
So the Arithmetic mean will be
Hence the AP is 10.
Can we insert two or more numbers between the two given numbers to form an Arithmetic progression?
Let A1 , A2 , A3 , …, An be n numbers between a and b such that a, A1 , A2 , A3 , …,An , b is an A.P.
Here, b is the (n + 2) th term, i.e., b = a + [(n + 2) – 1] d = a + (n + 1) d.
This gives
Thus, n numbers between a and b are as follows:
Example
Insert 4 numbers between 3 and 18 such that the resulting sequence is an A.P.
Solution:
Let A1, A2, A3 and A4 be the four numbers between 3 and 18 such that
3, A1, A2, A3, A4, 18
is the sequence in A.P.
Here, a = 3, b = 18, n = 6.
Therefore,
18 = 3 + (6 –1) d
15 = 5d
Thus A 1 = a + d = 3 + 3 = 6;
A 2 = a + 2d = 3 + 2 × 3 = 9;
A 3 = a + 3d = 3 + 3 × 3 = 12;
A 4 = a + 4d = 3 + 4 × 3 = 15
Hence, four numbers between 3 and 18 are 6, 9, 12 and 15
Geometric Mean
Geometric mean is a special type of average of two numbers. If we have two numbers then we will multiply the numbers and then take the square root of it.
If a and b are the two numbers then the geometric mean will be
Example
What is the geometric mean of 3 and 27?
Given
a = 3 and b = 27
Here we can see that the sequence 3, 9, 27 is a geometric progression.
How to calculate the Geometric mean of more than two numbers?
We can calculate the geometric mean of more than two numbers also. For calculating the geometric mean we have to multiply all the numbers and then take the nth root of that number.i.e. If we are multiplying two numbers, we are taking the square root, as we had taken in the above example.
If we will multiply three numbers then we will take the cube root. Likewise, if we are multiplying n number of terms then we will take the nth root of the number.
GM = n√ (a1 × a2 × ... × an)
Example
What is the Geometric Mean of 1, 4, 16, 64 and 256?
Solution:
Given
a1 = 1
a2 = 4
a3 =16
a4 = 64
a5 = 256
First we will multiply the given numbers
1 × 4 × 16 × 64 × 256 = 1048576
Then take the 5th root ( that is, the nth root where n = 4)
5√1048576 = 16
Geometric Mean = 5√ (1 × 4 × 16 × 64 × 256) = 16
Can we insert two or more numbers between the two given numbers to form a Geometric progression?
Let G 1, G2 ,…, G n be n numbers between positive numbers a and b such that
a, G 1, G 2, G 3,…, G n, b is a G.P.
Here, b is the (n + 2) th term, that is,
Thus, n numbers between a and b is as follows:
Example
Insert 3 numbers between 1 and 81 such that the resulting sequence is a G.P.
Solution:
Let G 1, G2 and G3 ,be the three numbers between 1 and 81 such that
1, G 1, G2 , G3 , 81
the sequence is in G.P.
Here, a5 = 81
Hence r5 = 81 that is, r = 3
G2 = ar2 = 1.32 = 9
G3 = ar3 = 1.33 = 27
Hence, three numbers between 1 and 81 are 3, 9 and 27 which in turn are making a Geometric sequence.
1, 3, 9, 27, 81
Relationship between A.M. and G.M.
As we have seen above the formula for the Arithmetic mean and the Geometric mean are as follows:
where a and b are the two given positive numbers.
Let A and G be A.M. and G.M.
So,
Now let’s subtract the two of them
This shows that A ≥ G
Example
Find the two numbers, If Arithmetic mean and Geometric mean of two positive real numbers are 20 and 16, respectively.
Solution:
Given
Now we will put these values of a and b in
(a – b)2 = (a + b)2 – 4ab
(a – b)2 = (40)2 – 4(256)
= 1600 – 1024
a – b = ± 24 ( by taking the square root) …(3)
By solving (1) and (3), we get
a + b = 40
a – b = 24
a = 8, b = 32 or a = 32, b = 8
Properties of relationship of A.M and G.M.
Property I: If the Arithmetic Mean and Geometric Mean of two positive numbers a and b are A and G respectively, then
A > G
As A – G > 0
Proof:
Let A and G be the Arithmetic Means and Geometric Means respectively of two positive numbers a and b.
Then,
As, a and b are positive numbers, it is obvious that A > G when G = -√ab.
Now we have to show that A ≥ G when G = +√ab.
We have,
Therefore, A - G ≥ 0 or, A ≥ G.
This proves that the Arithmetic Mean of two positive numbers can never be less than their Geometric Means.
Property II: If A be the Arithmetic Mean and G be the Geometric Mean between two positive numbers a and b, then the quadratic equation whose roots are a, b is
x2 - 2Ax + G2 = 0.
Proof:
As, A and G are the Arithmetic Mean and Geometric Mean respectively of two positive numbers a and b then, we have
The equation having a, b as its roots is
Property III: If A is the Arithmetic Means and G be the Geometric Means between two positive numbers, and then the numbers are A ± √A2 – G2
Proof:
Since, A and G be the Arithmetic Means and Geometric Means respectively then, the equation having its roots as the given numbers is
Example
Find two positive numbers whose Arithmetic Means increased by 2 than Geometric Means and their difference is 12.
Solution:
Let the two numbers be a and b. Then,
a-b = 12 ........................ (i)
Given
Now, a - b = 12
Solving (ii) and (iii), we get a = 16, b = 4
Hence, the required numbers are 16 and 4.
Example
If 34 and 16 are the Arithmetic Means and Geometric Means of two positive numbers respectively. Find the numbers.
Solution:
Let the two given positive numbers are a and b.
Given
Arithmetic Mean = 34
Geometric Mean = 16
As we know that
(a-b)2 = (a+b)2 – 4ab
⇒ (a-b)2 = (68)2 - 4 × 256 = 3600 ( from equation (i) and (ii))
⇒ a-b = 60............................... (iii)
After solving (i) and (iii), we get the numbers a = 64 and b = 4.
Hence, the required numbers are 64 and 4.
More Readings