Pair of Straight Lines

The equation ax² + 2hxy + by² + 2gx + 2fy + c = 0. Represents a second degree equation where a, h, b doesn’t variables simultaneously.

Let a ≠ 0.

Now, the above equation becomes

a² x² + 2ax (hy + g) = aby² – 2afy – ac

on completing the square on the left side, we get,

a² x² + 2ax (hy + g) = y² (h² – ab) + 2y (gh – af) + g² – ac.

i.e.    (ax + hy + g) = + √y²(h²–ab)+2y(gh–af)g²–ac

We cannot obtain x in terms of y, involving only terms of the first degree, unless the quantity under the radical sign be a perfect square. The condition for this is,

(gh – af)² = (h² – ab) (g² – ac)

i.e. g²h² – 2afgh + a²f² = g²h² – abg² – abg² – ach² + a2bc

cancelling and diving by a, we have the required condition

abc + 2fgh – af² – af² – bg² – ch² = 0

Illustration:

What is the point of intersection of two straight lines given by general equation ax²+ 2hxy + by² + 2gx + 2fy + c = 0?

Solution:

The general solution is

ax² + 2hxy + by² + 2gx + 2fy + c = 0                   …… (1)

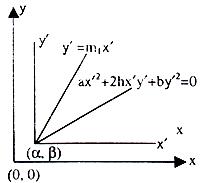
Let (α, ß) be the point of intersection we consider line paralleled transformation.

x = x’ + α,         y = y’ + ß

From (1) we have

a(x’ + α)² + 2h(x’ + α) (y’ + ß) + b(y’ + ß)² + 2g(x’ + α) + 2f(y’ + ß) + c = 0

⇒     ax’² + 2hx’y’ + by’² + a α² + 2hαß + bß² + 2gα + 2fß + 2x’(a α + hß + g) + 2y’ + 2y’ (hα + bß + f) = 0

⇒     ax’² + 2hx’y’ + by’² + 2x’(aα + hß + g) + 2g’ + 2y’ (hα + bß + f) = 0

Which must be in the form

ax'² + 2hx’y’ + by’ = 0

This cannot be possible unless

aα + hß + g = 0

hα + bß + f = 0

Solving

α/hf–bg = ß/hg–af = 1/ab–h²

α = hf–bg/ab–b², ß = hg–af/ab–h²

Illustration:

Represent lines y = 2x and y = 3x by a homogeneous equation of second degree

Solution:

(y – 2x) (y – 3x) = 0

Or 6x² – 5xy + y² = 0
 

Illustration:

Represent lines parallel to y = 2x and y = 3x by a second degree equation

Solution:

(y – 2x – c₁) (y – 3x – c₂) (where c₁ and c₂ are constants)

= 6x² – 5xy + y² + (3c₁ + 2c₂) x + (– c₁ – c₂) y + c₁ c₂ = 0

Note:

1. Homogeneous part is same as for the equation of above illustration. Therefore, the homogeneous part of a general second degree equation determines the slope of the lines i.e. lines parallel to ax² + 2hxy + by² + c = 0 and through the origin are represented by the equation ax² + 2hxy + by² = 0

2. The equation ax² + 2hxy + by² + 2fy + c = 0 represents a pair of parallel straight lines if h/a = b/h = f/g or bg² = af²

The distance between them is given by 2√g²–ac/a(a+b) or √f²–bc/b(a+b)

Illustration:

Does the second degree equation x² + 3xy + 2y² – x – 4y – 6 = 0 represents a pair of lines. If yes, find their point of intersection.

Solution:

We observe that

a = 1, h = 3/2, b = 2, g = –1/2, f = 2, c = – 6

abc + 2fgh – af² – bg² – ch² = – 12 + 3 – 4 – 1/2 + 27/2 = 0

Therefore the given second-degree equation represents a pair of lines, x² + 3xy + 2y² – x – 4y – 6 = (x + 2y + 2) (x + y – 3).

Consider the equations formed by first two rows of .

i.e. ax + hy + g = 0 and hx + by + f = 0

i.e. x + 3/2 y – 1/2 and 3/2 x + 2y – 2 = 0

Solving these, we get the required point of intersection.

i.e. 2x + 3y – 1 = 0

3x – 4y – 4 = 0

Solving the above equation, we get x = 8, y = –5.

Note:

(2x + 3y – 1)(3x + 4y – 4) ≠ x² + 3xy + 2y² – x – 4y – 6.

To read more, Buy study materials of Straight Lines comprising study notes, revision notes, video lectures, previous year solved questions etc. Also browse for more study materials on Mathematics here.