Progressive Wave
A progressive wave is defined as the onward transmission of the vibratory motion of a body in an elastic medium from one particle to the successive particle.
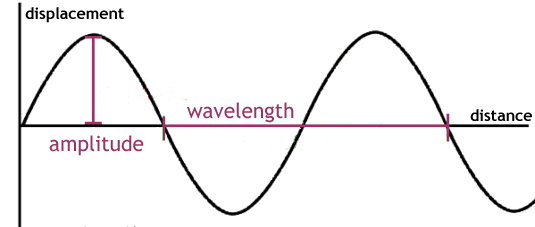
Equation of a plane progressive wave
An equation can be formed to represent generally the displacement of a vibrating particle in a medium through which a wave passes. Thus each particle of a progressive wave executes simple harmonic motion of the same period and amplitude differing in phase from each other.
Let us assume that a progressive wave travels from the origin O along the positive direction of X axis, from left to right (Fig. 7.6). The displacement of a particle at a given instant is
y = a sin ωt …... (1)
where a is the amplitude of the vibration of the particle and ω = 2πn.
The displacement of the particle P at a distance x from O at a given instant is given by,
y = a sin (ωt - φ) …... (2)
If the two particles are separated by a distance λ, they will differ by a phase of 2π. Therefore, the phase φ of the particle P at a distance
x is φ = (2π/λ) x
y = a sin (ωt - 2πx/λ) …... (3)
Since ω = 2πn = 2π (v/λ), the equation is given by,
y = a sin [(2πvt/λ) - (2πx/λ)]
y = a sin 2π/λ (vt – x) …... (4)
Since, ω = 2π/T, the equation (3) can also be written as,
y = a sin 2π (t/T – x/λ) …... (5)
If the wave travels in opposite direction, the equation becomes,
y = a sin 2π (t/T + x/λ) …... (6)
(i) Variation of phase with time

The phase changes continuously with time at a constant distance.
At a given distance x from O let φ1 and φ2 be the phase of a particle at time t1 and t2 respectively.
φ1 = 2π (t1/T - x/λ)
φ2 = 2π (t2/T - x/λ)
φ2 – φ1 = 2π (t2/T – t1/T) = 2π/T (t2 – t1)
?φ = (2π/T) ?t
This is the phase change ?φ of a particle in time interval ?t. If ?t = T, ?φ = 2π. This shows that after a time period T, the phase of a particle becomes the same.
(ii) Variation of phase with distance
At a given time t phase changes periodically with distance x. Let φ1 and φ 2 be the phase of two particles at distance x1 and x2 respectively from the origin at a time t.
Then, φ1 = 2π (t/T - x1/λ)
φ2 = 2π (t/T - x2/λ)
So, φ2 – φ1 = – 2π/λ (x2 – x1)
Thus, ?φ = – 2π/λ (?x)
The negative sign indicates that the forward points lag in phase when the wave travels from left to right.
When ?x = λ, ?φ = 2π, the phase difference between two particles having a path difference λ is 2π.
Refer this video to know more about progressive wave.
Characteristics of progressive wave
(a) Each particle of the medium executes vibration about its mean position. The disturbance progresses onward from one particle to another.
(b) The particles of the medium vibrate with same amplitude about their mean positions.
(c) Each successive particle of the medium performs a motion similar to that of its predecessor along the propagation of the wave, but later in time.
(d) The phase of every particle changes from 0 to 2π.
(e) No particle remains permanently at rest. Twice during each vibration, the particles are momentarily at rest at extreme positions, different particles attain the position at different time.
(f) Transverse progressive waves are characterised by crests and troughs. Longitudinal waves are characterised by compressions and rarefactions.
(g) There is a transfer of energy across the medium in the direction of propagation of progressive wave.
(h) All the particles have the same maximum velocity when they pass through the mean position.
(i) The displacement, velocity and acceleration of the particle separated by mλ are the same, where m is an integer.
Intensity and sound level
If we hear the sound produced by violin, flute or harmonium, we get a pleasing sensation in the ear, whereas the sound produced by a gun, horn of a motor car etc. produce unpleasant sensation in the ear.
The loudness of a sound depends on intensity of sound wave and sensitivity of the ear.
The intensity is defined as the amount of energy crossing per unit area per unit time perpendicular to the direction of propagation of the wave.
Intensity is measured in W m–2.
The intensity of sound depends on (i) Amplitude of the source (I α a2), (ii) Surface area of the source (I α A), (iii) Density of the medium (I α ρ), (iv) Frequency of the source (I α n2) and (v) Distance of the observer from the source (I α 1/r2 ).
The lowest intensity of sound that can be perceived by the human ear is called threshold of hearing. It is denoted by Io.
For sound of frequency 1 KHz, Io =10–12 W m–2. The level of sound intensity is measured in decibel. According to Weber-Fechner law,
decibel level (β) = 10 log10 [I/I0]
where Io is taken as 10–12 W m–2 which corresponds to the lowest sound intensity that can be heard. Its level is 0 dB. I is the maximum intensity that an ear can tolerate which is 1W m–2 equal to 120 dB.
β = 10 log10 (1/10-12)
β = 10 log10 (1012)
β = 120 dB
Progressive waves |
Stationary waves |
|
The disturbance produced in the medium travels onward, it being handed over from one particle to the next. Each particle executes the same type of vibration as the preceding one, though not at the same time. |
There is no onward motion of the disturbance as no particle transfers its motion to the next. Each particle has its own characteristic vibration. |
|
The amplitude of each partide is the same but the phase changes continuously, |
The amplitudes of the different particles are different, ranging from zero at the nodes to maximum at the antinodes. All the particles in a given segment vibrate in phase but in opposite phase relative to the particles in the adjacent segment. |
|
No particle is parmanently at rest. Different particles attain the state of momentary rest at different instants, |
The particles at the nodes are permanently at rest but other particles attain their position of momentary rest simultaneously. |
|
All the particles attain the same maximum velocity when they pass through their mean positions. |
All the particles attain their own maximum velocity at the same time when they pass through their mean positions. |
|
In the case of a longitudinal progressive wave all the parts of the medium undergo similar variation of density one after the other. At every point there will be a density variation. |
In the case of a longitudinal stationary wave the variation of density is different at different points being maximum at the nodes and zero at the antinodes. |
|
There is a flow of energy across every plane in the direction of propagation. |
Energy is not transported across any plane. |
|
|
Problem (JEE Main):
Two sound waves have intensities of 10 and 500µW/cm2. How many decibels is the second sound louder than the first?
(a) 7 dB (b) 1.7 dB
(c) 2.7 dB (d) 3.7 dB
Solution:
L1 = 10 log (10/I0)
and L2 = 10 log (500/I0)
Thus, L2 – L1 = 10 log (500/10) = 1.7 dB
Thus from the above observation, we conclude that, option (b) is correct.


Question 1
The displacement y of a wave traveling in the X-direction is given by y = 10–4sin (600t–2x + π/3) metres. where x is expressed in metres and t in seconds. The speed of the wave motion in ms–1 is
(a)200 (b) 300 (c) 600 (d) 1200
Question 2
A traveling wave in a stretched string is described by the equation, y = A sin(kx– ωt). The maximum particle velocity is
(a) Aω (b) ω/k (c) dω/dk (d) x/t
Question 3
Sound travels fastest in?
(a) vacuum (b) steel
(c) air (d) water
Question 4
A progressive Wave of frequency 500 Hz is traveling With a speed of 350 m/s. A compressional maximum appears at a place at a given instant. The minimum time interval after which a rarefaction maximum occurs at the same place is?
(a) 1/1000 s (b) 1/250 s
(c) 1/500 s (d) 1/350 s
Question 5
Sound whose frequency is 50 Hz
(a) has a relatively wavelength
(b) is very loud
(c) is very intense
(d) has a relatively short wavelength


| Q.1 | Q.2 | Q.3 | Q.4 | Q.5 |
|
b |
a |
b |
a |
a |
Related Resources:-
-
You might like to refer mechanical wave motion.
-
For getting an idea of the type of questions asked, refer the Previous Year Question Papers.
-
Click here to refer the most Useful Books of Physics.
- To get answer to any question related to progressive wave click here.
To read more, Buy study material of Waves comprising study notes, revision notes, video lectures, previous year solved questions etc. Also browse for more study materials on Physics here.
View courses by askIITians


Design classes One-on-One in your own way with Top IITians/Medical Professionals
Click Here Know More

Complete Self Study Package designed by Industry Leading Experts
Click Here Know More

Live 1-1 coding classes to unleash the Creator in your Child
Click Here Know More

a Complete All-in-One Study package Fully Loaded inside a Tablet!
Click Here Know MoreAsk a Doubt
Get your questions answered by the expert for free

