Chapter 3: Pair of Linear Equations in Two Variables Exercise – 3.2

Question: 1

Solve the following of equation graphically

x + y = 3

2x + 5y = 12

Solution:

2x + 5y = 12

We have,

x + y = 3

When y = 0 we have x = 3

When x = 0 we have y = 3

Thus we have the following table giving points on the line x + y = 3

X	0	3
Y	3	0

Now, 2 + 5y = 12

When x = 1, we have

Thus we have the following table giving points on the line 2x + 5y = 12

X	1	-4
Y	2	4

 Graph of the equation x + y = 3 and 2x + 5y = 12 is

Clearly two lines intersect at a point P (1, 2)

Hence x = 1 and y = 2

 

Question: 2

Solve the following of equation graphically

x - 2y = 5

2x + 3y = 10

Solution:

We have, x - 2y = 5 and 2x + 3y = 10

Now, x - 2y = 5

= x = 5 + 2y

When y = 0 then, x = 5

When y = -2 then, x = 1

Thus, we have the following table giving points on the line x - 2y = 5

X	5	- 1
Y	0	- 2

Now,  2x + 3y = 10

When y = 0, then x = 5

When y = 2, then x = 2 Thus, we have the following table giving points on the line 2x + 3y = 10

X	5	2
Y	0	2

 Graph of the equation x - 2y = 5 and 2x + 3y = 10

Clearly, two lines intersect at a point P (5, 0)

Hence x = 5 and y = 0

 

Question: 3

Solve the following of equation graphically

3x + y + 1 = 0

2x - 3y + 8 = 0

Solution:

We have, 3x + y + 1 = 0 and 2x - 3y + 8 = 0

Now 3x + y + 1 = 0

= y = -1-3x

When x = 0 then, x = -1

When y = -1 then, x = 2

Thus, we have the following table giving points on the line x - 2y = 5

X	-1	0
Y	2	-1

Now,  2x - 3y + 8 = 0

When y = 0, then x = -4

When y = 2, then x = 1 Thus, we have the following table giving points on the line 2x + 3y = 10

X	- 4	-1
Y	0	-2

Graph of the equation 3x + y + 1 = 0 and 2x - 3y + 8 = 0

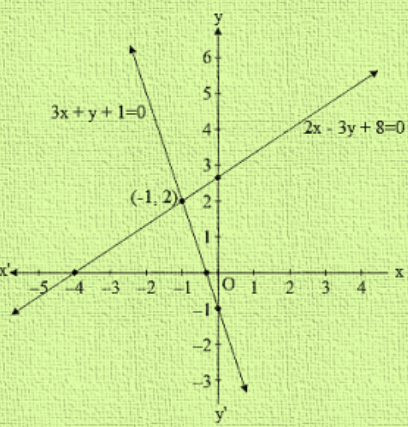
Clearly two lines intersect at a point P (-1, 2)

Hence x = -1 and y = 2

 

Question: 4

2x + y - 3 = 0

2x - 3y - 7 = 0

Solution:

We have, 2x + y - 3 = 0 and 2x - 3y - 7 = 0

Now 2x + y - 3 = 0

= y = 3 - 2x

When x = 0 then, x = 3

When x = 1 then, x = 1

Thus, we have the following table giving points on the line 2x + y - 3 = 0

X	0	1
Y	3	1

Now,   2x - 3y - 7 = 0

When x = 0, then y = 1

When x = 2, then y = -1 Thus, we have the following table giving points on the line 2x + 3y = 10

X	2	5
Y	-1	1

Graph of the equation 2x + y - 3 = 0 and 2x - 3y - 7 = 0

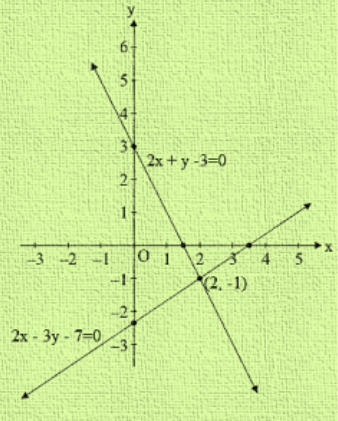
Clearly two lines intersect at a point P (2,-1)

Hence x = 2 and y = -1

 

Question: 5

x + y = 6

x - y = 2

Solution:

We have, x + y = 6 and x - y = 2

Now x + y = 6

= y = 6 - x

When x = 2 then, y = 4

When x = 3 then, y = 3

Thus, we have the following table giving points on the line x + y = 6

X	2	3
Y	4	3

Now ,  x - y = 2

= y = x – 2

When x = 0, then y = – 2

When x = 2, then y = 0 Thus, we have the following table giving points on the line 2x + 3y = 10

X	0	2
Y	2	0

Graph of the equation x + y = 6 and x - y = 2

Clearly two lines intersect at a point P (4, 2)

Hence x = 4 and y = 2

 

Question: 6

x - 2y = 6

3x - 6y = 0

Solution:

We have,   x - 2y = 6 and 3x - 6y = 0

Now x - 2y = 6

= x = 6 + 2y

When y = -2 then, x = 2

When y = -3 then, x = 0

Thus, we have the following table giving points on the line x - 2y = 6

X	2	0
Y	-2	-3

Now,  3x - 6y = 0

= x = 2y

When y = 0, then y = 0

When y = -1, then x = 2

Thus, we have the following table giving points on the line 3x - 6y = 0

X	0	2
Y	0	1

Graph of the equation x - 2y = 6 and 3x - 6y = 0

Clearly two lines are parallel to each other. So, the two lines have no common point. Hence the given system has no solutions.

 

Question: 7

Solve the following of equation graphically

x + y = 4

2x - 3y = 3

Solution:

We have,   x + y = 4 and 2x - 3y = 3

Now x + y = 4

= x = 4 - y

When y = 0 then, x = 4

When y = 2 then, x = 2

Thus, we have the following table giving points on the line x + y = 4

X	4	2
Y	0	2

Now ,  2x - 3y = 3

When y = 1, then x = 3

When y = -1, then x = 0

Thus, we have the following table giving points on the line 2x - 3y = 3

X	3	0
Y	1	-1

Graph of the equation x + y = 4 and 2x - 3y = 3

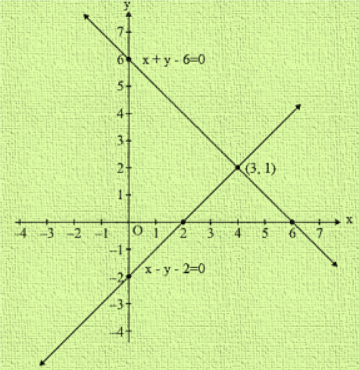
Clearly two lines intersect at a point P(3,1)

Hence x = 3 and y = 1

 

Question: 8

Solve the following of equation graphically

2x + 3y = 4

 x - y + 3 = 0

Solution:

So we have, 2x + 3y = 4 and x - y + 3 = 0

Now 2x + 3y = 4

When y = 0 then, x = 2

When y = 2 then, x = – 1

Thus, we have the following table giving points on the line x + y = 4

X	-1	2
Y	2	0

Now,  x - y + 3 = 0

= x = y - 3

When y = 3, then x = 0

When y = 4, then x = 1

Thus, we have the following table giving points on the line x - y + 3 = 0

X	0	1
Y	3	4

Graph of the equation 2x + 3y = 4 and x - y + 3 = 0

Clearly two lines intersect at (-1, 2)

Hence x = -1 and y = 2 is the solution of the given system of equations.

 

Question: 9

Solve the following of equation graphically

2x - 3y + 13 = 0

3x - 2y + 12 = 0

Solution:

So we have 2x - 3y + 13 = 0 and 3x - 2y + 12 = 0

Now,  2x - 3y + 13 = 0

When y = 1 then, x = – 5

When y = 3 then, x = – 2

Thus, we have the following table giving points on the line 2x - 3y + 13 = 0

X	- 5	- 2
Y	1	3

Now,  3x - 2y + 12 = 0

When y = 0, then x = -14

When y = 3, then x = -2

Thus, we have the following table giving points on the line x - y + 3 = 0

X	- 4	- 2
Y	0	3

Graph of the equation 2x - 3y + 14 = 0 and 3x - 2y + 12 = 0

Clearly two lines intersect at (- 2, 3)

Hence x = – 2 and y = 3 is the solution of the given system of equations.

 

Question: 10

Solve the following of equation graphically

2x + 3y + 5 = 0

3x + 2y - 12 = 0

Solution:

So we have 2x + 3y + 5 = 0 and 3x + 2y - 12 = 0

Now,  2x + 3y + 5 = 0

When y = 1 then, x = – 4

When y = -1 then, x = -1

Thus, we have the following table giving points on the line 2x + 3y + 5 = 0

X	-4	-1
Y	1	-1

Now,  3x + 2y - 12 = 0

When y = 0, then x = 4

When y = 3, then x = 6

Thus, we have the following table giving points on the line 3x - 2y - 12 = 0

X	4	6
Y	0	3

Graph of the equation 2x + 3y + 5 = 0 and 3x + 2y - 12 = 0

two lines intersect at (2, 3)

Clearly

Hence x = 2 and y = 3 is the solution of the given system of equations.

 

Question: 11

Solve the following of equation graphically

2x + 3y = 6

4x + 6y = 12

Solution:

So we have 2x + 3y = 6 and 4x + 6y = 12

 

Now,  2x + 3y = 5

When y = 0 then, x = 3

When y = 2 then, x = 0

 

Thus, we have the following table giving points on the line 2x + 3y = 6

X	0	3
Y	2	0

Now,   4x + 6y = 12

When y = 0, then x = 3

When y = 2, then x = 0

Thus, we have the following table giving points on the line 4x + 6y = 12

X	0	3
Y	2	0

Graph of the equation 2x + 3y = 6 and 4x + 6y = 12

Thus the graphs of the two equations are coincident.

Hence, the system of equations has infinitely many solutions.

 

Question: 12

Solve the following of equation graphically

x - 2y = 5

3x - 6y = 15

Solution:

So we have x - 2y = 5 and 3x - 6y = 15

Now,  x - 2y = 5

= x = 2y + 5

When y = -1 then, x = 3

When y = 0 then, x = 5

Thus, we have the following table giving points on the line x - 2y = 5

X	3	5
Y	1	0

Now,  3x - 6y = 15

When y = -2, then x = 1

When y = -3, then x = -1

Thus, we have the following table giving points on the line 3x - 6y = 15

X	1	-1
Y	-2	-3

 

Question: 13

Solve the following of equation graphically

3x + y = 8

6x + 2y = 16

Solution:

So we have 3x + y = 8 and 6x + 2y = 16

Now, x - 2y = 5

= y = 8 - 3x

When x = 2 then, y = 2

When x = 3 then, y = -1

Thus, we have the following table giving points on the line 3x + y = 8

X	2	3
Y	2	-1

Now,  6x + 2y = 16

When x = 1, then y = 5

When x = 3, then y = -1

Thus, we have the following table giving points on the line 6x + 2y = 16

X	1	3
Y	5	-1

Graph of the given equation

Thus, the graphs of the two equations are coincident

Hence, the system of equations has infinitely many solutions.

 

Question: 14

Solve the following of equation graphically

X - 2y + 11 = 0

3x + 6y + 33 = 0

Solution:

So we have x - 2y + 11 = 0 and 3x + 6y + 33 = 0

Now,  x - 2y + 11 = 0

= x = 2y -11

When y = 5 then, x = -1

When y = 4 then, x = -3

Thus, we have the following table giving points on the line x - 2y + 11 = 0

X	-1	-3
Y	5	-4

Now,   3x - 6y + 33 = 0

When y = 6, then x = -1

When y = 5, then x = -1

Thus, we have the following table giving points on the line 3x - 6y + 33 = 0

X	1	-1
Y	6	5

 

Thus, the graphs of the two equations are coincident

Hence, the system of equations has infinitely many solutions.

 

Question: 15

Solve the following of equation graphically

3x - 5y = 20

6x - 10y = – 40

Solution:

So we have 3x - 5y = 20 and 6x - 10y = – 40

Now, 3x -  5y = 20

When y = -1 then, x = 5

When y = – 4 then, x = 0

Thus, we have the following table giving points on the line 3x - 5y = 20

X	5	0
Y	-1	-4

Now,   6x - 10y = – 40

When y = 4, then x = 0

When y = 1, then x = – 5

Thus, we have the following table giving points on the line 6x - 10y = – 40

X	0	- 5
Y	4	1

Clearly, there is no common point between these two lines.

Hence, given systems of equations is in consistent.

 

Question: 16

Solve the following of equation graphically

x - 2y = 6

3x - 6y = 0

Solution:

So we have x - 2y = 6 and 3x - 6y = 0

Now,  x - 2y = 6

= x = 6 + 2y

When y = 0 then, x = 6

When y = -2 then, x = 2

Thus, we have the following table giving points on the line x - 2y = 6

X	6	2
Y	0	- 2

Now,    3x - 6y = 0

= x = 2y

When y = 0, then x = 0

When y = 1, then x = 2

Thus, we have the following table giving points on the line 3x - 6y = 0

X	0	2
Y	0	1

 

We find the lines represented by equations x- 2y = 6 and 3x – 6y = 0 are parallel. So, the two lines have no common point.

Hence, the given system of equations is in-consistent.

 

Question: 17

Solve the following of equation graphically

2y - x = 9

6y - 3x = 21

Solution:

So we have 2y - x = 9 and 6y - 3x = 21

Now,  2y - x = 9

= x = -9 + 2y

When y = 3 then, x = -3

When y = 4 then, x = -1

Thus, we have the following table giving points on the line 2y - x = 9

X	-3	-1
Y	3	4

Now,    6y - 3x = 21

= x = 2y - 7

When y = 2, then x = -3

When y = 3, then x = -1

Thus, we have the following table giving points on the line 6y - 3x = 21

X	-3	-1
Y	2	3

Graph of the given equation

We find the lines represented by equations 2y - x = 9 and 6y - 3x = 21 are parallel. So, the two lines have no common point.

Hence, the given system of equations is in-consistent.

 

Question: 18

Solve the following of equation graphically

3x - 4y - 1 = 0

Solution:

So we have 3x - 4y – 1 = 0 and 

Now, 

 3x - 4y -1 = 0

When y = 2 then, x = 3

When y = -1 then, x = -1

Thus, we have the following table giving points on the line 3x - 4y - 1 = 0

X	-1	3
Y	-1	2

Now,

When y = 0, then x = – 2.5

When y = 3, then x = 1.5

Thus, we have the following table giving points on the line 

X	- 2.5	1.5
Y	0	3

Graph of the given equation

We find the lines represented by equations 3x - 4y - 1 = 0 andare parallel. So, the two lines have no common point. Hence, the given system of equations is in-consistent.

 

Question: 19

Determine graphically the vertices of the triangle, the equations of whose sides are given below,

(i) 2y - x = 8, 5y - x = 14 and y - 2x = 1

(ii) y = x, y = 0 and 3x + 3y = 10

Solution:

(i)  2y - x = 8

5y - x = 14

y - 2x = 1

Now, 2y - x = 8

x = 2y - 8

When y = 2 then x = – 4

When y = 4 then x = 0

Thus, we have the following table giving points on the line 2y - x = 8

X	-4	0
y	2	4

Now, 5y - x = 14

x = 5y - 14

When y = 2, then x = 1

When y = 3 , then x = 1

Thus, we have the following table giving points on the line 5y – x = 14

X	- 4	1
y	2	3

We have,

y - 2x = 1

When y = – 1, then x = 1

When y = 3, then x = 1

Thus, we have the following table giving points on the line y - 2x = 1

X	-1	1
y	1	3

The graph of the given equation is:

From the graph of the lines represent by the given equation, we observe that the lines taken in pairs intersect at points A (- 4, 2) B(1, 3) and C(2, 5)

Hence the vertices of the triangle are A(- 4, 2) B(1, 3) and C(2, 5)

(ii) The given systems of equations are:

y = x

y = 0

3x + 3y = 10

We have, y = x

When x = 1, then y = 1

When x = -2, then y = - 2

Thus, we have the following table giving points on the line y = x

X	1	-2
y	3/7	3/4

The graph of the given equation is

From the graph of the lines represent by the given equation, we observe that the lines taken in pairs intersect at points

Hence the vertices of the triangle are

 

Question: 20

Determine graphically whether the system of equations x - 2y = 2, 4x - 2y = 5 is consistent or in-consistent

Solution:

x - 2y = 2

4x - 2y = 5

Now, x - 2y = 2

⟹ x = 2 + 2y

When y = 0 then, x = 2

When y = -1 then, x = 0

Thus, we have the following table giving points on the line x - 2y = 2

X	2	0
Y	0	-1

Now,  4x - 2y = 5

When y = 0, then x = 5/4

When y = 1, then x = 7/4

Thus, we have the following table giving points on the line 4x – 2y = 5

X	5/4	7/4
Y	0	1

Graph of the given equation

 

Clearly, the two lines intersect at (1, 0)

Hence, the system of equation is consistent.

 

Question: 21

Determine by drawing graphs, whether the following system of linear equation has a unique solution or not:

(i) 2x - 3y = 6 and x + y = 1

(ii) 2y = 4x - 6 and 2x = y + 3

Solution:

(i) 2x - 3y = 6 and x + y = 1

Now, 2x - 3y = 6

When y = 0 then, x = 3

When y = – 2 then, x = 0

Thus, we have the following table giving points on the line 2x - 3y = 6

X	3	0
Y	0	-2

Now,    x + y = 1

⟹ x = 1 - y

When y = 0, then x = 1

When y = 1, then x = 0

Thus, we have the following table giving points on the line x + y = 1

X	0	1
Y	1	0

Graph of the given equations:

(ii) 2y = 4x - 6

2x = y + 3

Now, 2y = 4x - 6

When y = -1 then, x = 1

When y = 5 then, x = 4

Thus, we have the following table giving points on the line 2y = 4x - 6

X	1	4
Y	-1	5

Now,  2x = y + 3

⟹ x = (y + 3)/2

When y = 1, then x = 2

When y = 3, then x = 3

Thus, we have the following table giving points on the line 2x = y + 3

X	2	3
Y	1	3

Graph of the given equations:

We find the graphs of the two equations are consistent. Therefore, the system of equations has infinitely many solutions.

 

Question: 22

Solve graphically each of the following system of linear equations. Also, find the coordinates of the points where the lines meet axis of y.

(i) 2x - 5y + 4 = 0 and 2x + y - 8 = 0

(ii) 3x + 2y = 12 and 5x - 2y = 4

(ii) 2x + y - 11 = 0 and x - y - 1 = 0

(iv) x + 2y - 7 = 0 and 2x - y - 4 = 0

(v) 3x + y - 5 = 0 and 2x - y - 5 = 0

(vi) 2x - y - 5 = 0 and x - y - 3 = 0

Solution:

(i) 2x - 5y + 4 = 0 and 2x + y - 8 = 0

Now, 2x - 5y + 4 = 0

When y = 2 then, x = 3

When y = 4 then, x = 8

Thus, we have the following table giving points on the line 2x - 5y + 4 = 0

X	3	8
Y	2	4

Now,   2x + y - 8 = 0

When y = 4, then x = 2

When y = 2, then x = 3

Thus, we have the following table giving points on the line 2x = y + 3

X	3	8
Y	2	4

 

Clearly, two intersect at P(3,2)

Hence, x = 3 and y = 2 is the solution of the given system of equations.

We also observe that the lines represented by 2x - 5y + 4 = 0 and 2x + y - 8 = 0 meet y-axis at A(0, 4/5)  and B(0, 8) respectively.

(ii) 3x + 2y = 12 and 5x - 2y = 4

Now, 3x + 2y = 12

When y = 3 then, x = 2

When y = -3 then, x = 6

Thus, we have the following table giving points on the line 3x + 2y = 12

X	2	6
Y	3	-3

Now,  5x - 2y = 4

When y = 3, then x = 2

When y = -7, then x = -2

Thus, we have the following table giving points on the line 5x - 2y = 4

X	2	-2
Y	3	-7

 

Clearly, two intersect at P(2, 3)

Hence, x = 2 and y = 3 is the solution of the given system of equations.

We also observe that the lines represented by 3x + 2y = 12 and 5x - 2y = 4 meet y-axis at A(0, 6) and B(0,-2) respectively.

(iii) 2x + y - 11 = 0 and x - y - 1 = 0

Now, 2x + y = 11

y = 11 - 2x

When y = 4 then, x = 3

When y = – 5 then, x = 1

Thus, we have the following table giving points on the line 2x + y = 11

X	4	5
Y	3	1

Now,   x - y = 1

= y = x - 1

When x = 2, then y = 1

When y = 3, then y = 2

Thus, we have the following table giving points on the line x - y = 1

X	2	3
Y	1	2

Clearly, two intersect at P (4, 3)

Hence, x = 4 and y = 3 is the solution of the given system of equations.

We also observe that the lines represented by 2x + y = 11 and x - y = 1 meet y-axis at A(0, 11)  and B(0,-1) respectively.

(iv) x + 2y - 7 = 0

2x - y - 4 = 0

Sol:

Now, 2x - y - 4 = 0

X = 7 - 2y

When y = 1 then, x = 5

When y = -2 then, x = 3

Thus, we have the following table giving points on the line 2x + y = 11

X	5	3
Y	1	2

Now,   2x - y - 4 = 0

= y = 2x - 4

When x = 2, then y = 0

When y = 0, then y = -4

Thus, we have the following table giving points on the line 2x - y - 4 = 0

X	2	0
Y	0	- 4

 

Clearly, two intersect at P (3, 2)

Hence, x = 3 and y = 2 is the solution of the given system of equations.

We also observe that the lines meet y-axis at A(0, 3.5)  and B(0,- 4) respectively.

(v) 3x + y - 5 = 0 and 2x - y - 5 = 0

Solution

Now, 3x + y - 5 = 0

y = 5 - 3x

When x = 1 then, y = 2

When x = 2 then, y = -1

Thus, we have the following table giving points on the line 3x + y – 5 = 0

X	1	2
Y	2	-1

Now,  2x - y - 5 = 0

= y = 2x - 5

When x = 0, then y = -5

When x = 2, then y = -1

Thus, we have the following table giving points on the line 2x - y - 5 = 0

X	0	2
Y	- 5	- 1

Clearly, two intersect at P (2,-1)

Hence, x = 2 and y = -1 is the solution of the given system of equations.

We also observe that the lines meet y-axis at A(0, 5)  and B(0, – 5) respectively.

(vi)

The given equations are

2x - y - 5 = 0   ….(ii)

x - y - 3 = 0   ….(ii)

The two points satisfying (i) can be listed in a table as,

X	1	3
Y	- 3	1

The two points satisfying (ii) can be listed in a table as,

X	1	5
Y	-2	-2

Now, graph of equations (i) and (ii) can be drawn as,

It is seen that the solution of the given system of equation is given by x = 2, y = -1.

Also, it is observed that the lines (i) and (ii) meet the y-axis at the points (0, – 3) and (0, – 5) respectively.

 

Question: 23

Solve the following system of linear equations graphically and shade the region between the two lines and x- axis.

(i) 2x + 3y = 12 and x - y = 1

(ii) 3x + 2y - 4 = 0 and 2x - 3y - 7 = 0

(iii) 3x + 2y - 11 = 0 and 2x - 3y + 10 = 0

Solution:

(i) 2x + 3y = 12 and x - y = 1

The system of the given equation is y = x, 3y = x and y + x = 8

Now, 2x + 3y = 12

When y = 2, then x = 3

When y = 4, then x = 0

Thus, we have the following table:

X	0	3
Y	4	2

We have,

x - y = 1

x = y + 1

When y = 0, then x = 1

When y = 1, then x = 2

Thus, we have the following table:

X	1	2
Y	0	1

Graph of the given system is:

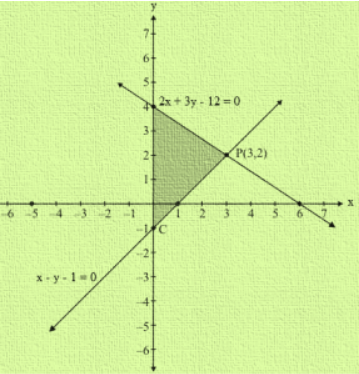
Clearly the two lines intersect at P (3, 2)

Hence x = 3 and y = 2 is the solution of the given system of equations.

(ii) 3x + 2y - 4 = 0 and 2x - 3y - 7 = 0

The system of the given equation is 3x + 2y - 4 = 0 and 2x - 3y - 7 = 0

Now, 3x + 2y - 4 = 0

When y = 5, then x = - 2

When y = 8, then x = - 4

Thus, we have the following table:

X	- 2	- 4
Y	5	8

We have,

2x - 3y - 7 = 0

When y = 1, then x = 5

When y = -1, then x = 2

Thus, we have the following table:

X	5	2
Y	1	-1

Graph of the given system is:

Clearly the two lines intersect at P(2,-1)

Hence x = 2 and y = -1 is the solution of the given system of equations.

(iii) 3x + 2y - 11 = 0 and 2x - 3y + 10 = 0

Now, 3x + 2y - 11 = 0

When y = 1, then x = - 3

When y = 4, then x = 1

Thus, we have the following table:

X	3	1
Y	1	4

We have,

2x - 3y + 10 = 0

When y = 0, then x = - 5

When y = 2, then x = - 2

Thus, we have the following table:

X	- 5	- 2
Y	0	2

Graph of the given system is:

Clearly the two lines intersect at P(1, 4)

Hence x = 1 and y = 4 is the solution of the given system of equations.

 

Question: 24

Draw the graphs of the following equations on the same graph paper:

2x + 3y = 12 and x - y = 1

Solution:

Now, 2x + 3y = 12

When y = 0, then x = 6

When y = 2, then x = 3

Thus, we have the following table:

X	6	3
Y	0	2

We have,

x - y = 1

x = 1 + y

When y = 0, then x = 1

When y = -1, then x = 0

Thus, we have the following table:

X	1	0
Y	0	-1

Graph of the given system is:

Clearly the two lines intersect at A (3, 2)

We also observe that the lines meet y-axis B (0, - 1) and C (0, 4)

Hence the vertices of the required triangle are A (3, 2) , B (0,-1) and C (0, 4).

 

Question: 25

Draw the graphs of x - y + 1 = 0 and 3x + 2y - 12 = 0. Determine the coordinates of the vertices of the triangle formed by these lines and x-axis and shade the triangular area. Calculate the area bounded by these lines and x- axis.

Solution:

The given system of equations is:

x - y + 1 = 0 and 3x + 2y - 12 = 0

Now, x - y + 1 = 0

x = y - 1

When y = 3, then x = 2

When y = -1, then x = -2

Thus, we have the following table:

X	2	- 2
Y	3	- 1

We have,

3x + 2y - 12 = 0

When y = 6, then x = 0

When y = 3, then x = 2

Thus, we have the following table:

X	0	2
Y	6	3

Graph of the given system is:

Clearly, the two lines intersect at A(2, 3)

We also observe that the lines meet x-axis B(-1, 0) and C(4, 0)

Thus x = 2 and y = 3 is the solution of the given system of equations.

AD is drawn perpendicular A on x-axis. Clearly we have,

AD = y-coordinate point A (2, 3)

AD = 3 and BC = 4- ( – 1) = 4 + 1 = 5

 

Question: 26

Solve graphically the system of linear equation:

4x - 3y + 4 = 0 and 4x + 3y - 20 = 0

Find the area bounded by these lines and x-axis.

Solution:

The given system of equation is 4x - 3y + 4 = 0 and 4x + 3y - 20 = 0

Now, 4x - 3y + 4 = 0

When y = 0, then x = -1

When y = 4, then x = 2

Thus, we have the following table:

X	2	-1
Y	4	0

We have,

4x + 3y - 2 = 0

When y = 0, then x = 5

When y = 4, then x = 2

Thus, we have the following table:

X	5	2
Y	0	4

Graph of the given system is:

Clearly, the two lines intersect at A (2, 4)

We also observe that the lines meet x-axis B (-1, 0) and C(5, 0)

Thus x = 2 and y = 4 is the solution of the given system of equations.

AD is drawn perpendicular A on x-axis. Clearly we have,

AD = y-coordinate point A (2, 4)

AD = 3 and BC = 5 – (-1) = 4 + 1 = 6

Area of the shaded region =1/2 × base × altitude

=1/2 × 6 × 4

= 12 sq. units

 

Question: 27

Solve the following system of linear equations graphically:

3x + y - 11 = 0 and x - y - 1 = 0

Shade the region bounded by these lines and y- axis. Also find the area of the region bounded by these lines and y-axis.

Solution:

The given system of equations is 3x + y - 11 = 0 and x - y - 1 = 0

Now, 3x + y - 11 = 0

y = 11 - 3x

When x = 0, then y = 11

When x = 3, then y = 2

Thus, we have the following table:

X	0	3
Y	11	2

We have

x - y - 1 = 0

y = x - 1

When x = 0, then y = -1

When x = 3, then y = 2

Thus, we have the following table:

X	0	3
Y	-1	2

Graph of the given system is:

Clearly, the two lines intersect at A (3, 2)

We also observe that the lines meet y-axis B(0,11) and C (0, – 1)

Thus x = 3 and y = 2 is the solution of the given system of equations.

AD is drawn perpendicular A on x-axis. Clearly we have,

AD = y-coordinate point A(2, 4)

AD = 3 and BC = 11- (- 1) = 11 + 1 = 12

Area of the shaded region = 1/2 × base × altitude

= 1/2 × 12 × 3

= 18 sq. units

 

Question: 28

Draw the graph of the following equation:

2x - 3y + 6 = 0

2x + 3y - 18 = 0

y - 2 = 0

Solution:

Now, 2x - 3y + 6 = 0

When y = 0, then x = -3

When y = 2, then x = 0

Thus, we have the following table:

X	- 3	0
Y	0	2

We have

2x + 3y - 18 = 0

When y = 2, then x = 6

When y = 6, then x = 0

Thus, we have the following table:

X	6	0
Y	2	6

We have

y - 2 = 0

y = -2

Graph of the given system of equations:

From the graph of three equations, we find that the three lines taken in pairs intersect each other at points A(3, 4) , B(0, 2) and C(6, 2)

Hence, the vertices of the required triangle are (3, 4) , (0, 2) and (6, 2)

From the graph, we have

AD = 4 - 2 = 2

BC = 6 - 0 = +

Area of the shaded region = 1/2 × base × altitude

= 1/2 × 6 × 2

= 6 sq. units

 

Question: 29

Solve the following system of equations graphically:

2x - 3y + 6 = 0 and 2x + 3y - 18 = 0

Also, find the area of the region bounded by these lines and y-axis.

Solution:

The given system of equations:

2x - 3y + 6 = 0 and 2x + 3y - 18 = 0

Now, 2x - 3y + 6 = 0

When x = 0, then y = 2

When x = -3, then y = 0

Thus, we have the following table:

X	0	-3
Y	2	6

We have

2x + 3y - 18 = 0

When y = 2, then x = 6

When y = 6, then x = 0

Thus, we have the following table:

X	6	0
Y	2	6

Graph of the given system of equations:

Clearly, the two lines intersect at A (3, 4) .Hence x = 3 and y = 4 is the solution of the given system of equations.

From the graph, we have

AD = x-coordinate point A(3, 4) = 3

BC = 6 - 2 = 4

Area of the shaded region =1/2 × base × altitude

= 1/2 × 4 × 3

= 6 sq. units

 

Question: 30

Solve the following system of linear equation graphically;

4x - 5y - 20 = 0 and 3x + 5y - 15 = 0

Solution:

Now, 4x - 5y - 20 = 0

When y = 0, then x = 5

When y = – 4, then x = 0

Thus, we have the following table:

X	5	0
Y	0	-4

We have

3x + 5y - 15 = 0

When y = 0, then x = 5

When y = - 4, then x = 0

Thus, we have the following table:

X	5	0
Y	0	3

Graph of the given system of equations:

Clearly, the two lines intersect at A(5, 0). Hence x - 5 , y - 0 is the solution of the given system of equations.

The lines meet y-axis at B(0, -4) and C(0, 3) respectively.

The vertices of the triangle are (5, 0) , (0,- 4) and (0, 3)

 

Question: 31

Draw the graphs of the equations 5x - y = 5 and 3x - y = 3. Determine the coordinates of the vertices of the triangle forms by these lines and y-axis. Calculate the area of the triangle forms.

Solution:

5x - y = 5

⟹ y = 5x - 5

Three solutions of this equation can be written as follows:

X	0	1	2
y	-5	0	5

3x - y = 3

Y = 3x - 3

X	0	1	2
y	- 3	0	3

The graphical representation of the two lines will be as follows:

It can observed that the required triangle is ABC

The coordinates of its vertices A (1, 0) B(0, - 3) and C(0, -5)

 

Question: 32

Form the pair of linear equation in the following problems, and find their solution graphically:

(i) 10 students of class X took part in mathematics quiz. If the number of girls is 4 more than the number of boys. Find the number of boys and girls who took part in the quiz.

(ii) 5 pencils and 7 pens together costs Rs.50, whereas 7 pencils and 5 pens together cost Rs.46.

Find the cost of one pencil and one pen.

(iii) Champa went to a sale to purchase some pants and skirts. When her friends asked her how many of each kind she had bought, she answered, “the number of skirts is two less than twice the number of pants purchase”. Also, ” the number of skirts is four less than four times the number of pants purchased.”Help her friends to find how many pants and skirts champa bought.

Solution:

(i) Let the number of girls and boys in the class be x and y respectively.

According to the Q.,

x + y = 10 and x - y = 4 are the given equations

Now, x + y = 10

x = 10 - y

Three solutions of this equation can be written as follows:

X	4	5	6
Y	6	5	4

x - y = 4

x = 4 + y

Three solutions of this equation can be written as follows:

X	5	4	3
Y	1	0	-1

The graphical representation is as follows:

From the graph, it can be observed that the two lines intersect each other at point (7, 3).

So x = 7 and y = 3

The number of girls and boys in the class are 7 and 3 respectively.

(ii) Let the cost of one pencil and one pen Rs. x and Rs. y respectively.

According to the Q., we have,

5x + 7y = 50

7x + 5y = 50

Now, 5x + 7y = 50

Three solutions of this equation can be written as follows:

X	3	10	- 4
y	5	0	10

7x + 5y = 46

Three solutions of this equation can be written as follows:

X	8	3	-2
y	-2	5	12

The graphical representation is as follows:

From the graph it can be observed that the two lines intersect each other at the point (3, 5)

So x = 3 and y = 5

Therefore the cost of the pencil and the pen are 3 and 5 respectively.

(iii) Let us denote the number of pants by x and the number of skirts be y. Then the equations formed are :

y = 2x – 2     …… (i)

y = 4x - 2   ….. (ii)

The graphs of the equations (i) and (ii) can be drawn by finding two solutions for each of the equations. They are given in the following table.

X	2	0
Y=  2x - 2	2	-2

Hence, the graphic representation is as follows.

The two lines inetersect at the point (1, 0). So, x = 1, y = 0 is the required solution of the pair of linear equations, i.e., the number of parts she purchased is 1 and she didi not buy any skirt.

 

Question: 33

Solve the following system of equations graphically:

Shade the region between the lines and y-axis

(i) 3x - 4y = 7 and 5x + 2y = 3

(ii) 4x - y = 4 and 3x + 2y = 14

Solution:

(i) 3x - 4y = 7 and 5x + 2y = 3

The given system of linear equation is 3x - 4y = 7 and 5x + 2y = 3

Now, 3x - 4y = 7

When x = 1 then, y = – 1

When x = – 3 then y = – 4

Thus, we have the following table

X	1	- 3
Y	-1	- 4

Now, 5x + 2y = 3

When x = 1 then, y = – 1

When x = 3 then y = – 6

Thus, we have the following table

X	1	3
Y	-1	- 6

Graph of the given system of equations are:

Clearly the two lines intersect at A(1,-1)

Hence x = 1 and y = -1 is the solution of the given system of equations.

(ii) 4x - y = 4 and 3x + 2y = 14

The given system of linear equation is 4x - y = 4 and 3x + 2y = 14

Now, 4x - y = 4

y = 4x - 4

When x = 0 then, y = – 4

When x = -1 then y = - 8

Thus, we have the following table

X	0	-1
y	- 4	-8

Now, 3x + 2y = 14

When x = 0 then, y = 7

When x = 4 then y = 1

Thus, we have the following table

X	0	4
Y	7	1

Graph of the given system of equations are:

Clearly the two lines intersect at A (2, 4)

Hence x = 2 and y = 4 is the solution of the given system of equations.

 

Question: 34

Represent the following pair of equations graphically and write the coordinates of points where the lines intersects y-axis

x + 3y = 6 and 2x - 3y = 12

Solution:

The given systems of equations are:

x + 3y = 6 and 2x - 3y = 12

Now, x + 3y = 6

When x = 0 then, y = 2

When x = 3 then y = 1

Thus, we have the following table

X	0	3
Y	2	1

Now, 2x - 3y = 12

When x = 0 then, y = – 4

When x = 6 then y = 0

Thus, we have the following table

X	0	6
Y	-4	0

Graph of the given system of equations are:

Clearly the two lines meet y-axis at B(0, 2) and C(0, -4) respectively.

Hence the required coordinates are (0, 2) and (0, -4)

 

Question: 35

Given the linear equation 2x + 3y - 8 = 0, write another in two variables in two variables such that the geometrical representation of the pair so formed is (i) intersecting lines (ii) parallel lines (iii) coincident lines

Solution:

(i) For the two lines a₁x + b₁x + c₁ = 0 and a₂x + b₂x + c₂ = 0 to be intersecting. We must have

So the other linear equation can be 5x + 6y - 16 = 0

(ii) For the two lines a₁x + b₁x + c₁ = 0 and a₂x + b₂x + c₂ = 0 to be parallel we must have

So, the other linear equation can be 6x + 9y + 24 = 0

(iii) For the two lines a₁x + b₁x + c₁ = 0  and a₂x + b₂x + c₂ = 0 to be coincident we must have

So, the other linear equation can be 6x + 9y + 24 = 0