Maths Revision Notes on Circles for IIT JEE

The circle is an important concept in geometry that you must have studied in Class 9, Class 10 and Class 11. Many questions in competitive exams and engineering entrance tests include questions from the Circles chapter. This is why askIITians experts have compiled revision notes for Circles to help you revise all the concepts, theorems, and formulae related to Circles in no time. These notes include all the important topics based on the engineering entrance exams syllabus. With these notes, you can revise concepts related to circles for JEE, NEET, CBSE board exams and more.

The main topics covered in these online revision notes for Circles are the equation of the circle, equation of the chord, basic properties of circles, length of the chord of a circle, properties of chords of a circle, tangents to a circle, properties of tangents to a circle, the radical axis of circles, and equations of the family of circles. You can also check Maths Notes for Class 9, 10, 11, 12 separately on our website if you want to practice every concept thoroughly.

Online Revision Notes for Circles

The equation of a circle with its centre at C(x0, y0) and radius r is: (x – x0)2 + (y – y0)2 = r2
If x0 = y0 = 0 (i.e. the centre of the circle is at origin) then the equation of the circle reduces to x2 + y2 = r2.
If r = 0 then the circle represents a point or a point circle.
The equation x2 + y2 + 2gx + 2fy + c = 0 is the general equation of a circle with centre (–g, –f) and radius √(g2+f2-c).
Equation of the circle with points P(x1, y1) and Q(x2, y2) as extremities of a diameter is (x – x1) (x – x2) + (y – y1)(y – y2) = 0.
For a general circle, the equation of the chord is x1x + y1y + g(x1 + x) + f(y1 +y) + c = 0
For circle x2 + y2 = a2, the equation of the chord is x1x + y1y = a2
The equation of the chord AB
(A ≡ (R cos α, R sin α); B ≡ (R cos β, R sin β)) of the circle x2 + y2 = R2 is given by x cos ((α + β )/2) + y sin ((α - β )/2) = a cos ((α - β )/2)
Chords are equidistant from the centre of a circle if and only if they are equal in length.

Equal chords of a circle subtend equal angles at the centre

The angle subtended by an arc at the centre is double the angle subtended by the same arc at the circumference of the circle.
The angle between the tangent and the radius is 90°.
Angles in the same segment are equal.
The angle in a semicircle is 90°.
Two angles at the circumference subtended by the same arc are equal.

The below table describes the equations of a circle according to changes in radii and centres:

The point P(x1, y1) lies outside, on, or inside a circle.

S ≡ x2 + y2 + 2gx + 2fy + c = 0, according as S1 ≡ x12 + y12 + 2gx1 + 2fy1 + c > = or < 0.

The equation of the chord of the circle x2 + y2 + 2gx + 2fy +c=0 with M(x1, y1) as the midpoint of the chord is given by:

xx1 + yy1 + g(x + x1) + f(y + y1) = x12 + y12 + 2gx1 + 2fy1 i.e. T = S1

In case the radius and the central angle of a triangle are given, the length of the chord can be computed using the formula

Length of the chord = 2r sin (c/2), where ‘c’ is the central angle and ‘r’ is the radius

If a circle has two secants QR and ST, then

If there is a circle that has one tangent and one secant, then the square of the tangent is equal to the product of the secant segment and its external segment.
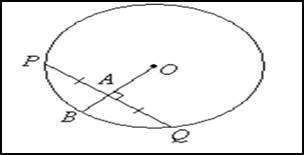
If a radius or the diameter of a circle is perpendicular to a chord, then it divides the chord into two equal parts. The converse also holds true.

Hence, in the below figure, if OB is perpendicular to PQ, then PA = AQ.

The perpendicular bisector of a chord passes through the centre of a circle. In the figure given below, OA is the perpendicular bisector of chord PQ and it passes through the circle. Similarly, OB is the perpendicular bisector of RS and it also passes through the centre.

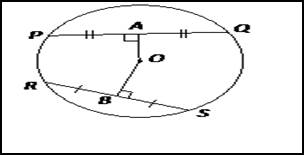
In the above circle, OA is the perpendicular bisector of the chord PQ and it passes through the centre of the circle. OB is the perpendicular bisector of the chord RS and it passes through the centre of the circle.
If two chords in a circle are congruent, then they are equidistant from the centre.
If two chords are equidistant from the centre, then they are congruent. So, in the figure, if PQ = RS then OA = OB and similarly if OA = OB then PQ = RS.

The arcs intercepted by two congruent chords in a circle are congruent.
If two arcs are congruent, then their corresponding chords are congruent.
If a line y = mx + c intersects the circle x2 + y2 = a2 in two distinct points A and B then length of intercept AB = 2√[(a2 (1+m2)-c2)/ (1+m2)]
The equation of the tangent on a point on the circle and the equations of the chord of contact are both represented by T = 0. But the difference between the two is that in the case of the chord of contact, the point say (x1, y1) lies outside the circle while in the case of tangent it lies on the circle.
Common tangents may be either direct or transverse.
Direct common tangents to two circles meet on the line joining the centres and hence divide them externally in the ratio of their radii.
Transverse common tangents also meet on the line of centres as the direct common tangents but the difference is that they divide it internally in the ratio of their radii.

There cannot be any common tangent if a circle lies completely inside another without touching it.
If two circles touch each other internally, one and only one common tangent is possible.
If two circles touch each other in two distinct points, then in such a case, two common tangents can be drawn.
In the case of two circles touching each other externally, three common tangents can be drawn.
Four common tangents are possible when two circles neither touch nor intersect but one lies completely inside the other.
How to calculate direct common tangents:

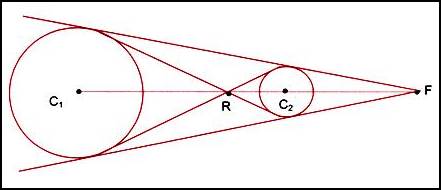
Suppose there are two circles with centres at C1 and C2 and radii as r1and r2 and P is the point of intersection of direct common tangents as shown in the figure below:

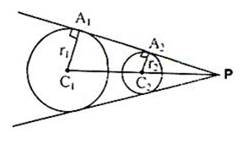
C1A1, C2A2 are perpendiculars from C1 and C2 to one of the tangents. The point ‘P’ divides C1C2 externally in the ratio r1: r2. So, to find the direct common tangent we just need to find the point P which divides the line joining the centre externally in the ratio of radii.

The equation of direct common tangent is SS1 = T2 where S is the equation of one circle.

Length of C1C2 > |r1 – r2|
For two circles with equations S and S’, where

S ≡ x2 + y2 + 2gx + 2fy + c and

S’ ≡ x2 + y2 + 2g’x + 2fy’ + c’

Then equation of radical axis of two circles S = 0 and S’ = 0 is given by S = S’

2(g – g’)x + 2(f – f’)y + (c – c’) = 0

The condition for two circles with centres at C1(x1, y1) and C2(x2, y2) and radii r1, r2 respectively, to touch each other internally and externally a

Internally: If |C1 C2| = |r2 – r1| and the point of contact is ((r1x2-r2x1)/(r1+r2) , (r1y2-r2y1)/(r1+r2)).
Externally: If |C1 C2| = |r2 – r1| and the point of contact is ((r1x2+r2x1)/(r1+r2) , (r1y2+r2y1)/(r1+r2)).

For the circle S = 0, the equation, S – S’ = 0 represents the equation of the radical axis i.e. the equation of radical axis is

2x(g – g’) + 2y(f – f’) + c – c’ = 0

If the circles S = 0 and S’ = 0 intersect in real and distinct points, then S – S’ = 0 is the equation of the common chord of the two circles.

If S’ = 0 and S = 0 touch each other, then S – S’ = 0 is the equation of the common tangent to the two circles at the point of contact.

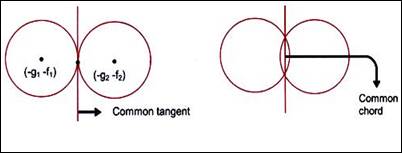
The radical axis of the two circles is perpendicular to the line joining the centres.

The radical axes of three circles taken two at a time are concurrent and the point of concurrency is known as the radical centre.
The radical axes of two circles bisect their direct common tangents.
If two circles cut the third circle orthogonally, then the radical axis of the two circles will pass through the centre of the third circle.
If in a system of circles, every two of them have the same radical axis, then the system is a coaxial system

The equations of the family of circles in various cases:

1. S1 + λ S2 = 0 is the family of circles passing through the intersection of S1 = 0 and S2 = 0.
2. The family of circles passing through the intersection of line L and circle S is given by S + λL = 0. A particular value of λ in the equation yields a unique circle.
3. The family of circles passing through two given points A(x1, y1) and B(x2, y2) are given by the equation

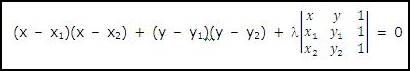
Two circles S = 0 and S1 = 0 with centres at (–g, –f) and (–g1, –f1) cut each other orthogonally if the following equation is satisfied:

2gg1+ 2ff1 = c + c

Revision Notes for Circles FAQs

Are circles important for JEE?

Circles is an important topic for JEE Main and Advanced. You can expect 1-2 questions based on circles in these examinations. So you must practice its concepts in school as well as in coaching classes. This will help you score higher percentile in the entrance tests.

What is included in NCERT Class 10 Circles Chapter?

You study all the basic concepts related to circles in Class 10 such as tangents to a circle, sector of a circle, the segment of a circle, etc. This knowledge is further applied in learning conic sections in Class 11.

Where can I find CBSE revision notes for Maths?

askIITians provides Class 6, 7, 8, 9, 10, 11, 12 notes for Maths in a chapter-wise format. You can refer to our online revision notes and practice all Maths concepts on your own.

How can I master the Maths subject for competitive exams?

Enrol in online Maths coaching for CBSE, JEE, NEET and other popular school boards and learn in live, interactive classes from our experts. We also provide dedicated study notes for all the students like chapter notes, NCERT solutions, video lectures, mind maps, flashcards, study planners and much more. All these resources can help you master the Maths subject for competitive exams.